751.11 Bearings

Elastomeric bearings are economical bearings. Typically the footprint of elastomeric bearings is rectangular or square; however, circular elastomeric bearings may be considered for skewed bridges or short span curved steel bridges with lower vertical loading, translational and rotational demands where multi-rotational (pot) bearings could be unnecessary overdesign. See Structural Project Manager or Structural Liaison Engineer before using circular elastomeric bearings. Elastomeric bearings may be either plain or laminated (steel reinforced) pads. There are three types of elastomeric bearings utilized by MoDOT based on pad type and function:

Plain fixed – integral bents only (Use laminated fixed if slope or grade dictates.)
Laminated fixed (Use girder chairs if minimum dead load, slope or grade dictates.)
Laminated expansion

PTFE Bearings

PTFE type bearings shall be used when laminated elastomeric expansion bearings are not capable of handling the required expansion length or the required total thickness of elastomer exceeds 5”.

Multi-Rotational High Load Bearings

Pot bearings can be considered for use on bridges when bearing design service reaction exceeds 300 kips or bearing area exceeds 15” x 24” and effects of curvature are considered. Due to high cost, limited use of these types of bearings are recommended. Consult Structural Project Manager before using Pot Bearings.

Girder/Beam Chairs

Chairs shall be used at integral bents for prestressed and steel girders and beams if slope requirements cannot be met by elastomeric laminated fixed bearings.

Chairs shall also be used for prestressed girders and beams at fixed intermediate bents where minimum dead load or slope requirements cannot be met by elastomeric laminated fixed bearings and under the smaller girder where girder sizes change.

Bearing Type Location

The following figure shows an example of what type of bearings should be used at the given locations.

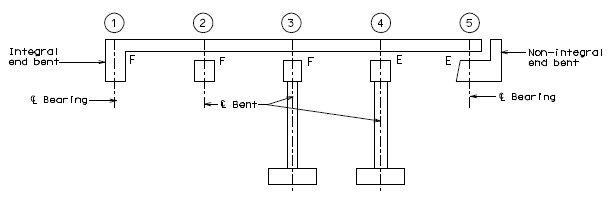
File:751.11 Bearing Type Location.gif

Integral construction (end bent typ.) – Use plain fixed elastomeric bearing or laminated fixed if necessary.
Flexible intermediate bent (pile cap) – Use laminated fixed bearings for flexible intermediate bents.
Rigid intermediate bent w/out thermal expansion – Use laminated fixed bearings at bents with no temperature movement.
Rigid intermediate bent w/ thermal expansion – Use laminated expansion bearings or PTFE/Pot bearings if necessary.
Rigid end bent w/ thermal expansion - Use laminated expansion bearings or PTFE/Pot bearings if necessary.

751.11.1.2 Limit States and Load Factors

Limit States and Load Factors

In general, each component shall satisfy the following equation:

$\,Q=\sum {n_{i}}\ {\gamma _{i}}\ {Q_{i}}\leq \phi {R_{n}}=R_{r}$

Where:

$\,Q$	= Total factored force effect
$\,Q_{i}$	= Force effect
$\,n_{i}$	= Load modifier
$\,\gamma _{i}$	= Load factor
$\,\phi$	= Resistance factor
$\,R_{n}$	= Nominal resistance
$\,R_{r}$	= Factored resistance

Limit States

The following limit states shall be considered for bearing design:

STRENGTH – I

SERVICE – I

See LRFD Table 3.4.1-1 and LRFD 3.42 for Loads and Load Factors applied to each given limit state.

Service loads without including dynamic load allowances shall be used for bearing design.

Resistance Factors

STRENGTH limit states, see LRFD Article 6.5.4.2 and 5.5.4.2

For all other limit states,

\,\phi

= 1.00

Load Modifiers

751.11.2 Design

751.11.2.1 Elastomeric Bearings

General

Elastomeric Bearing design shall follow AASHTO LRFD “Method A”.

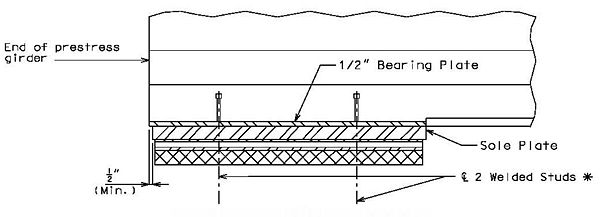
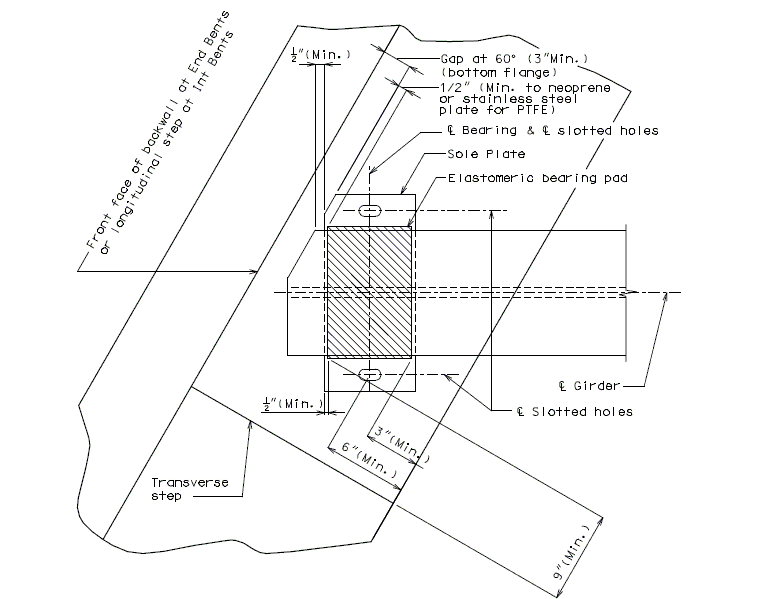
The elastomeric expansion bearings and fixed bearings for steel girders consist of a sole plate and elastomeric bearing pad. Elastomeric bearings at integral end bents and fixed bearings for prestressed girders at intermediate bents consist of elastomeric bearing pad without a sole plate.

The sole plates are flat or beveled to match the profile grade of the roadway surface along the centerline of the girder. If the profile grade is equal to or less than 0.01 or the total drop across the bearing is equal to or less than 1/8 inch, then a flat sole plate may be used and if necessary, elastomer thickness increased to address the bearing rotation due to the profile grade. Sole plates are used to anchor girders, ensure uniform distribution of the compressive stress and strain over bearing area and reduce dead load bearing rotations.

At fixed bearings, girder chairs may be considered as an alternate if roadway slope, rotation or bearing pressure is requiring tall or large bearing pads.

The elastomer bearing pad shall be 60 durometer hardness and reinforced with 1/8 inch steel shim plates when laminated pads are required by design.

When rectangular bearings are used, increased rotation bearing capacity can be achieved by orientating the pad with the shorter dimension of the pad parallel with the span of the girder.

Plain elastomeric bearing pads should be utilized where vertical loads, translations and rotations are relatively small. For integral end bents, use ½” fixed plain pads when taper due to girder slope or grade is less than 1/8”, or use a laminated bearing pad when taper equals or exceeds 1/8” due to girder slope or grade. In the rare circumstance when intermediate bents are made integral by extending the beam cap stirrups into the diaphragm, consideration can be given to utilizing ½” plain pads under similar conditions of slope.

Laminated elastomeric bearing pads should be utilized where there is greater need for vertical loading, translational and rotational capacity. For non-integral end bents, non-integral intermediate bents and for integral end bents when taper exceeds 1/8” due to girder slope or grade use laminated elastomeric bearing pads where the pad thickness and number of laminates is based on design that should account for larger vertical loads, translation, rotation and meet slope of girder and grade requirements.

Size Limitations

Use the values in the standard bearing pad tables if possible.

Bearing pad length shall be 8” minimum for MoDOT Standard Prestressed (P/S) I-Girders, Adjacent P/S Box Beams and Steel I-Girders.

Bearing pad length shall be 5” minimum for P/S NU Girders and P/S Spread Box Beams. Not preferred but for consideration of some lower bound limits as used by the Nebraska Department of Roads (NDOR) that developed the NU I-Girder, for P/S NU Girders only, and based on NDOR guidelines, a 4” minimum bearing pad length and/or 2 ft. minimum bearing pad width can be used with Structural Project Manager or Structural Liaison Engineer approval.

Plain Fixed for P/S I-Girder:
W, width of bearing ≤ Bottom flange width – 1.5”

Plain Fixed for steel girders:
Bottom flange width – 2” ≤ W ≤ Bottom flange width

Laminated Fixed for P/S I-Girder:
W = Bottom Flange width – 1.5”

Laminated Expansion Bearing Pads for PS I-Girders and Laminated Fixed and Expansion Pads for Steel Girders:
9” ≤ W ≤ Bottom flange width + 7”

Stability

The following requirement shall be met for ensuring stability of the bearing pad:

Rectangular Pads

$\,h_{rt}\leq MIN{\big \{}L/3,W/3{\big \}}$

Circular Pads

$\,h_{rt}\leq {D/4}$

Temperature Movement

Determining temperature movements for bearings requires the calculation of the thermal origin of the bridge. To accomplish this, the stiffness of each bent must be calculated. Once the thermal origin is established, the total temperature movement for each bearing location can be found by the following equations:

$\,\triangle _{s}=\gamma \alpha$ (temperature range)x(distance from thermal origin)(0.65)

Where:

$\,\triangle _{s}$	= maximum shear deformation of the pad
$\,\gamma$	= 1.2 for laminated pads
	= 1.0 for PTFE bearings
$\,\alpha$	= coefficient of thermal expansion
	= 0.0000065 IN/IN/ºF (steel superstructure)
	= 0.000006 IN/IN/ºF (concrete superstructure)
(0.65)	= 65% reduction due to forgiving nature of elastomer (LRFD 14.7.6.3.4)

temperature range	= 150ºF (steel superstructure)
	= 120ºF (concrete superstructure)

Shear Deformation

Both plain elastomeric and laminated elastomeric shall meet the following criteria for shear deformation.

$\,h_{rt}\geq \ 2\triangle _{s}$

Where:

$\,h_{rt}$ = total elastomer thickness, in.

The following table represents the available heights of laminated expansion pads that are required due to the shear deformation criteria. PTFE type bearings shall be used if $\,\triangle _{s}>2.5\ in.$

**Laminated Expansion Bearing Pad Heights**
Laminated Expansion Pads
$\,\triangle _{s}$ , in.	Interior layer thickness, in.	Exterior layer thickness, in.	$\,h_{rt}$ , in.	n	C, in.
1/2	1/2	1/4	1	2	1 1/4
3/4	1/2	1/4	1 1/2	3	1 7/8
1	1/2	1/4	2	4	2 1/2
1 1/4	1/2	1/4	2 1/2	5	3 1/8
1 1/2	1/2	1/4	3	6	3 3/4
1 3/4	1/2	1/4	3 1/2	7	4 3/8
2	1/2	1/4	4	8	5
2 1/4	1/2	1/4	4 1/2	9	5 5/8
2 1/2	1/2	1/4	5	10	6 1/4

Where:

C	= total thickness of bearing including steel shims, in.
n	= total number of interior layers of elastomer + 1*

* The additional “1” is accounting for the two exterior layers as per LRFD 14.7.5.3.5

Compressive Stress

Service loads without including dynamic load allowance shall be used for design checks.

At intermediate bents with 2 bearing pads per girder line (i.e. PS I-girders), use ½ of the live load reaction for each pad.

Total Load

Plain Elastomeric Pad

$\,\sigma _{TL}\leq \ 0.800ksi$

Laminated Elastomeric Pad

$\,\sigma _{TL}\leq 1.00GS$

and

$\,\sigma _{TL}\leq 1.0ksi$

Minimum Dead Load

$\,\sigma _{DLmin}\geq 0.200ksi$

The 200 psi minimum requirement is intended for preventing the horizontal crawling of the bearing when it is not attached to the top surface. This requirement has been applied to the bearing designs detailed in EPG 751 even though these bearings are detailed with positive attachment to the flange of the girder. Compliance with the requirement is desirable but is not mandatory if it results in a special bearing design or special superstructure treatments.

Where:

$\,\sigma _{TL}$	= compressive stress due to total load = $\,{\frac {DC+DW+LL}{L\times W}}$
$\,\sigma _{DLmax}$	=compressive stress due to maximum dead load = $\,{\frac {DC+DW}{L\times W}}$
$\,\sigma _{DLmin}$	=compressive stress due to minimum dead load = $\,{\frac {DC}{L\times W}}$
G	= shear modulus = 0.130 ksi for compressive stress calculations
S	= shape factor for thickest layer of elastomer = $\,{\frac {LW}{2h_{ri}(L+W)}}$
S	= shape factor for circular pads = $\,{\frac {D}{4h_{ri}}}$
$\,h_{ri}$	= thickness of the i^th elastomer layer, in.

Rotation

Rotations shall be taken as the maximum possible change in slope between the top and bottom surfaces of the bearing caused by the initial lack of parallelism between the bottom of girder flange and top of bearing and the girder end rotation due to imposed loads and movements. Rotations may be calculated by a straight-line approximation of dead and live load deflections taken at 1/10 or 1/4 points. The following equation must be satisfied to ensure that uplift does not occur under any combination of loads and corresponding rotation:

Rectangular Laminated Elastomeric Pad

$\,\sigma _{s}\geq \ 0.5GS\left({\frac {L}{h_{ri}}}\right)^{2}{\frac {\theta _{s}}{n}}$

Where:

L	= length of bearing pad in the direction of traffic
W	= width of bearing pad in the direction perpendicular to traffic
G	= shear modulus = 0.200 ksi for rotation calculations
$\,\theta _{s}$	= maximum rotation about the transverse axis due to initial lack of parallelism and total service load, rad
n	= total number of interior layers of elastomer + 1*

* The additional “1” is accounting for the two exterior layers as per LRFD 14.7.5.3.5

Plain elastomeric pads contained within integral concrete diaphragms are not subject to this rotation requirement.

This criteria is intended as an uplift check. If uplift is not possible at the bearing due to an integral diaphragm/beam at the abutment or an integral diaphragm/beam at an intermediate bent, then this criteria would not be applicable.

Circular Laminated Elastomeric Pad

$\,\sigma _{s}\geq \ 0.375GS\left({\frac {D}{h_{ri}}}\right)^{2}{\frac {\theta _{s}}{n}}$

Where:

D = diameter of pad

Compressive Deflection

Deflections of elastomeric bearings should be limited to ensure that deck joints and seals are not damaged. Also, bearings that are too flexible can produce a small step across a deck joint giving rise to a high impact loading when traffic passes from one girder to the other. The maximum relative deflection across a joint is suggested to be less than 1/8”.

Initial compressive deflection of plain elastomeric or in any layer of steel reinforced elastomeric bearing at the service limit state without impact shall not exceed $\,0.07h_{ri}$ .

Values for compressive strain can be calculated by using LRFD Figure C14.7.6.3.3-1 for 60 durometer reinforced bearings.

There is a code check for compressive deflection of a single layer but no code check for total compressive deflection.

Taper

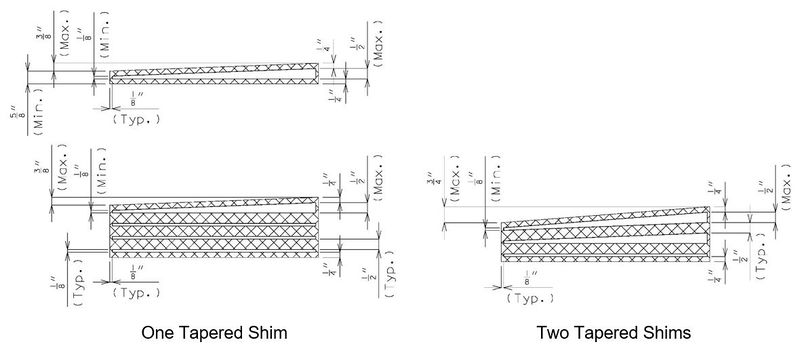
When the difference between the slope of the girder and the slope of a bearing pad exceeds 1/8” taper shall be considered. For laminated expansion pads for both PS I-Girders and steel girders and for laminated fixed pads for steel girders where sole plates are required, sole plates shall be tapered to account for the girder slope. Sole plates shall have a minimum thickness of 1 1/2” at the centerline of bearing, and a minimum thickness of 1” at the edge. Plain fixed pads shall not be tapered. At integral end bents where girder slope is greater than 1/8”, use laminated fixed pads. Laminated bearing taper is provided by tapering the top shim to match the slope of the girder to the nearest 1/8” total difference in thickness. Thickness of shims shall be a minimum of 1/8” and a maximum of 1/2”. For excessive girder slopes it may be necessary to taper the top two shims with a maximum combined taper of the bearing of 3/4 inch. Tapered layers of elastomer are not permitted.

Anchor Bolts

Check with Structural Project Manager or Liaison before using anchor bolts other than ASTM F1554. When anchor bolts are used for laminated fixed for steel girders or laminated expansion for steel and P/S I-girders, they should be designed for a minimum horizontal force equal to 25% of the maximum dead load applied to the bearing. With SPM approval for rehab superstructure job designer may design anchor bolt for a minimum horizontal force equal to 15% of the maximum dead load applied to the bearing. Designer may ignore live load in horizontal force computation. Anchor bolts shall be ASTM F1554 Grade 55 unless higher grade anchor bolts are required to meet design requirements. Grade 105 bolts shall not be used in applications where welding to the bolt is required. (Revise anchor bolt notes in EPG 751.50 Standard Detailing Notes for plans with different grade and nuts, e.g. “ASTM F1554 Grade 55” to “ASTM F1554 Grade 105” and “ASTM A563 Grade A Heavy Hex nuts” to “ASTM A563 Grade DH Heavy Hex nuts”.)

Bolt properties (Updated in 2022)
Bolt Type	Nominal Bolt Diameter (in.)	Min. Tensile Strength (ksi)	Min. Yield Strength (ksi)	Comments
ASTM F1554 (Grade 36)	½” to 4”	58	36	Preferred specification for structural supports anchored into concrete.
ASTM F1554 (Grade 55)	½” to 4”	75	55
ASTM F1554 (Grade 105)	½” to 3”	125	105
ASTM A449 Type 1 Type 3 (weathering)	¼” to 1”	120	92	Material properties are applicable for old A325 bolts (Pre-2016). May be manufactured as a threaded rod.
	over 1” to 1 ½”	105	81
	1 ¾” to 3”	90	58	Typically used for larger diameter headed anchors for bearings in girder shelves.
ASTM A307	¼” to 4”	60	NA	Typically used for regular strength steel connections. Should not be used for applications that require significant tensile or flexural forces on the bolt.
ASTM F3125 Grade A325 Type 1 Type 3 (weathering)	½” to 1 ½”	120	92	Typically used as high strength fasteners, but also used as headed anchors for bearings in girder shelves.
ASTM F3125 Grade A490 Type 1 Type 3 (weathering)	½” to 1 ½”	150	130	Typically used as high-strength fastener. Galvanization is not allowed.
Note: The above table is a comprehensive list for bolts typically used in structural applications on MoDOT projects. These values will aid designers when substitutions need to be made for similar design applications.

Anchor bolts are used on bearings with sole plates. For bridges that require seismic details only, design anchor bolts for flexure and shear induced from horizontal seismic forces and design for tension due to uplift forces if present. For bridges that require a seismic analysis, design anchor bolts for flexure induced from horizontal seismic forces and separately design for the effects of combined tension and shear.

Limit the number of bolts per bearing to four. Concrete Shear blocks shall be used when anchor bolts cannot be designed to resist earthquake loading. For shear blocks details for P/S girder see, EPG 751.22.3.13 Concrete Shear Blocks. Use similar details for shear blocks for steel girder.

Bearing details are shown in EPG 751.11.3 Details for two 1 ½”, 2” and 2 ½“ diameter anchor bolts. Consult Structural Project Manager before using bolt diameters larger than 2 1/2". Revise bearing details if four anchor bolts are required by design. For larger than 2 ½” diameter anchor bolt revise details in EPG 751.11.3.5 Anchor Bolts, bearing details and anchor bolt notes in EPG 751.50 Standard Detailing Notes.

For seismic details only (strength limit states)

Anchorage shall be adequate to resist lateral loads.

Horizontal factored shear force, ${F_{H}}={\sqrt {(F_{T})^{2}+(F_{L})^{2}}}$ in kips per girder

For expansion bearings, transverse F_T = 0.25(DL) per girder & longitudinal F_L = 0.

Where DL = unfactored dead load reaction at the bent, kips

For fixed bearings, transverse F_T = 0.25(DL) per girder and Longitudinal F_L = (0.25)(segment weight)/(# of girders)

Segment weight includes the full width of superstructure and should be distributed appropriately among fixed bents.

When centrifugal forces are present, they should be checked independently (not combined with seismic loads shown above or below). Use a 1.75 load factor with the centrifugal force and check resistance at the Strength Limit state as described below.

For complete seismic analysis

Anchor bolt designs must meet requirements for strength limit states from above as well as seismic forces from seismic analysis. Anchorage shall be adequate to resist lateral loads as well as uplift force from seismic analysis.

At Intermediate bent,

{F_{H}}={\frac {\sqrt {\sum {V_{L}}^{2}+\sum {V_{T}}^{2}}}{N_{G}}}

where: F_H = horizontal seismic force per girder, kips

If columns are designed for plastic hinging, use the plastic hinging shear.

$\sum {V_{L}}$ = summation of top of column longitudinal shears at the bent

$\sum {V_{T}}$ = summation of top of column transverse shears at the bent

N_G = number of girders at the bent

At end bents

Use the same formula as above, except substitute the abutment shears in place of the top of column shears.

{P_{u}}={\frac {F_{H}}{{n_{b}}R}}

P_u = horizontal factored shear force per bolt, kips

n_b = the number of bolts per girder

For intermediate bent, R = 1.0

For end bent, R = 1.0 for seismic category A & B and 0.8 for seismic category C & D.

Flexural Resistance

Factored flexural stress shall be less than or equal to the nominal flexural resistance. Assume the bolt is restrained from rotation by the sole plate, but free to translate.

M = P_u x L/2 = maximum moment per bolt, inch-kips

L = moment arm from center of sole plate to top of the beam cap, inches

S = section modulus for the bolt $={\frac {\pi D^{3}}{32}}$ , cubic inches.

D = minimum body diameter, inches. For F1554 bolts use D = 0.92 x nominal bolt diameter. Alternately, the minimum body diameter can be retrieved from ASTM F1554 Table 4. For all other bolt types the nominal bolt diameter may be used because the bolt is unthreaded in the flexural zone and the minimum body diameter is similar to the nominal diameter.

{f_{b}}={\frac {M}{S}}\leq {\emptyset _{f}}{F_{Y}}

Where:

∅_f = 1.0 resistance factor for seismic details only (strength limit states) and for complete seismic analysis

Yield strength of the anchor bolt, F_Y = 55 ksi for Grade 55 and F_Y = 105 ksi for Grade 105

Shear Resistance

Factored shear force shall be less than or equal to the nominal shear resistance.

{P_{u}}\leq {\emptyset _{s}}{R_{n}}

where:

∅_s = 0.75 resistance for seismic details only (strength limit states) and 1.0 for complete seismic analysis

Nominal shear resistance of an F1554 anchor bolt, R_n = 0.5A_bF_ubN_s LRFD 6.13.2.12-1, C6.13.2.7 and 14.8.3

Note: ASTM F1554 allows the body diameter of the bolt to be reduced to provide an area not less than the stress area of the threaded portion of the bolt. For this reason, there are no differences in calculation for threads beings included or excluded from the shear plane. If another type of bolt is used for any reason the nominal shear resistance should be determined from LRFD Eq. 6.13.2.7-1 or 2.

A_b $={\frac {\pi D^{2}}{4}}$ = nominal area of the anchor bolt, square inches

F_ub = minimum tensile strength of the anchor bolt, ksi

= 75 ksi for Grade 55, 125 ksi for Grade 105

N_s = number of shear plane per anchor bolt = 1

D = nominal bolt diameter, inches

Tensile Resistance

Factored tensile force shall be less than or equal to the nominal tensile resistance.

T = the maximum seismic tensile (uplift) force (DL ± EQ) per girder from the seismic analysis, kips. If (DL+EQ) and (DL-EQ) are both compressive, then there is no need to design the bolts for tensile force.

{\frac {T}{n_{b}}}\leq {\emptyset _{t}}{T_{n}}

Where:

∅_t = 0.8 resistance factor for seismic details only (strength limit states) and 1.0 for complete seismic analysis

n_b = the number of bolts per girder

Nominal tensile resistance of the anchor bolt, T_n = 0.76A_bF_ub LRFD 6.13.2.10.2-1 and 14.8.3

where:

A_b = nominal area of the anchor bolt, square inches

F_ub = minimum tensile strength of the anchor bolt, ksi

= 75 ksi for Grade 55, 125 ksi for Grade 105

Combined Tension and Shear Resistance

The resistance of anchor bolts for combined tension and shear force shall be determined in accordance with LRFD 6.13.2.11.

If ${\frac {P_{u}}{R_{n}}}\leq 0.33$ , then T_n = 0.76A_bF_ub LRFD 6.13.2.11-1

Otherwise

{T_{n}}=0.76{A_{b}}{F_{ub}}{\Big [}1-{\big (}{\frac {p_{u}}{{\emptyset _{s}}{R_{n}}}}{\big )}^{2}{\Big ]}^{0.5}

LRFD 6.13.2.11-2

Strength Limit States and Seismic Details only

Maximum horizontal factored shear force reaction, F_H for given anchor bolts and total thickness of bearing including steel shims:

	Max. shear deformation of the pad, ∆s, in.		1/4	1/2	3/4	1	1 1/4	1 1/2	1 3/4	2	2 1/4
	Total thick. of bearing including steel shims, C in.		1 1/4	1 7/8	2 1/2	3 1/8	3 3/4	4 3/8	5	5 5/8	6 1/4
Anchor Bolt Type	No. of Anchor Bolt	Nominal dia. of anchor bolt, in.	Maximum horizontal factored shear force reaction, F_H
ASTM F1554 Grade 55	2	1.5	28	22	17	15	13	11	10	9	8
		2	67	51	41	35	30	26	23	21	19
		2.5	131	100	81	68	58	51	46	41	38
	4	1.5	57	43	35	29	25	22	20	18	16
		2	135	103	83	69	60	53	47	42	38
		2.5	263	200	162	136	117	103	91	82	75
ASTM F1554 Grade 105	2	1.5	54	41	33	28	24	21	19	17	15
		2	128	98	79	66	57	50	45	40	37
		2.5	251	191	154	129	111	98	87	79	72
	4	1.5	108	83	67	56	48	42	38	34	31
		2	257	196	158	133	114	100	89	81	73
		2.5	502	382	309	259	223	196	174	157	143
Notes:	Calculations were based on 1-1/2" thick sole plate at centerline of bearing. Flexural resistance calculations were based on minimum body diameter of the anchor bolt. For centrifugal forces, check anchor bolts for separate load. For complete seismic analysis case, design anchor bolts as explained above.

751.11.2.2 Standard Pad Tables for P/S I-Girders

Standard Laminated Expansion Bearing Pads for Prestress I-Girders

Bottom Flange Width in.	L in.	W in.	S Int. Layer	S Ext.Layer	DL min kips	DLmax + LL kips	$\,\sigma _{TL}<ksi$
17	9	12	5.14	10.29	21.6	72.2	0.669
17	10	14	5.83	11.67	28.0	106.2	0.758
17	11	15.5	6.43	12.87	34.1	142.6	0.836
17	12	15.5	6.76	13.53	37.2	163.5	0.879
17	13	15.5	7.07	14.14	40.3	185.2	0.919
17	14	15.5	7.36	14.71	43.4	207.5	0.956
18	9	12	5.14	10.29	21.6	72.2	0.669
18	10	14	5.83	11.67	28.0	106.2	0.758
18	11	16	6.52	13.04	35.2	149.1	0.847
18	12	16.5	6.95	13.89	39.6	178.8	0.903
18	13	16.5	7.27	14.54	42.9	202.8	0.945
18	14	16.5	7.57	15.15	46.2	227.4	0.985
19	9	13	5.32	10.64	23.4	80.9	0.691
19	10	14	5.83	11.67	28.0	106.2	0.758
19	11	16	6.52	13.04	35.2	149.1	0.847
19	12	17.5	7.12	14.24	42.0	194.3	0.925
19	13	17.5	7.46	14.92	45.5	220.6	0.970
19	14	17.5	7.78	15.56	49.0	245.0	1.000
24	12	18	7.20	14.40	43.2	202.2	0.936
24	13	20	7.88	15.76	52.0	260.0	1.000
24	14	22	8.56	17.11	61.6	308.0	1.000
24	15	22.5	9.00	18.00	67.5	337.5	1.000
24	16	22.5	9.35	18.70	72.0	360.0	1.000
24	17	22.5	9.68	19.37	76.5	382.5	1.000
24	18	22.5	10.00	20.00	81.0	405.0	1.000

Note:

Pads shown in table must be checked for:

Shear deformation, Stability, Rotation, and Compressive deflection.

Standard Laminated Fixed Bearing Pads for Prestress I-Girders

Bottom Flange Width in.	L in.	W in.	S Ext.Layer	DL min kips	DLmax + LL kips	$\,\sigma _{TL}<ksi$
17	8	15.5	10.55	24.8	124.0	1.000
17	9	15.5	11.39	27.9	139.5	1.000
17	10	15.5	12.16	31.0	155.0	1.000
17	11	15.5	12.87	34.1	170.5	1.000
17	12	15.5	13.53	37.2	186.0	1.000
17	13	15.5	14.14	40.3	201.5	1.000
17	14	15.5	14.71	43.4	217.0	1.000
18	8	16.5	10.78	26.4	132.0	1.000
18	9	16.5	11.65	29.7	148.5	1.000
18	10	16.5	12.45	33.0	165.0	1.000
18	11	16.5	13.20	36.3	181.5	1.000
18	12	16.5	13.89	39.6	198.0	1.000
18	13	16.5	14.54	42.9	214.5	1.000
18	14	16.5	15.15	46.2	231.0	1.000
19	8	17.5	10.98	28.0	140.0	1.000
19	9	17.5	11.89	31.5	157.5	1.000
19	10	17.5	12.73	35.0	175.0	1.000
19	11	17.5	13.51	38.5	192.5	1.000
19	12	17.5	14.24	42.0	210.0	1.000
19	13	17.5	14.92	45.5	227.5	1.000
19	14	17.5	15.56	49.0	245.0	1.000
24	8	22.5	11.80	36.0	180.0	1.000
24	9	22.5	12.86	40.5	202.5	1.000
24	10	22.5	13.85	45.0	225.0	1.000
24	11	22.5	14.78	49.5	247.5	1.000
24	12	22.5	15.65	54.0	270.0	1.000
24	13	22.5	16.48	58.5	292.5	1.000
24	14	22.5	17.26	63.0	315.0	1.000
24	15	22.5	18.00	67.5	337.5	1.000

Note:

Pads sizes in above tables (not at integral bents) must be checked for:
Shear deformation, Stability, Rotation, and Compressive deflection.

For pads at integral bents:
Shear deformation and Rotation need not be checked.

For fixed bearings requiring more than two layers of 1/4” elastomer separated by 1/8” shim, use shape factor calculated for the interior layer of elastomer in the laminated expansion bearing pad table.

Standard Plain Fixed Bearing Pads for Prestress I-Girders

Bottom Flange Width in.	L in.	W in.	S Single Layer	DL min kips	DLmax + LL kips	$\,\sigma _{TL}<ksi$
17	9	12	5.14	21.6	86.4	0.800
17	9	14	5.48	25.2	100.8	0.800
17	9	15.5	5.69	27.9	111.6	0.800
17	10	14	5.83	28.0	112.0	0.800
17	10	15.5	6.08	31.0	124.0	0.800
17	11	15.5	6.43	34.1	136.4	0.800
17	12	15.5	6.76	37.2	148.8	0.800
17	13	15.5	7.07	40.3	161.2	0.800
17	14	15.5	7.36	43.4	173.6	0.800
18	9	12	5.14	21.6	86.4	0.800
18	9	14	5.48	25.2	100.8	0.800
18	9	16.5	5.82	29.7	118.8	0.800
18	10	14	5.83	28.0	112.0	0.800
18	10	16.5	6.23	33.0	132.0	0.800
18	11	16	6.52	35.2	140.8	0.800
18	11	16.5	6.60	36.3	145.2	0.800
18	12	16.5	6.95	39.6	158.4	0.800
18	13	16.5	7.27	42.9	171.6	0.800
18	14	16.5	7.57	46.2	184.8	0.800
19	9	13	5.32	23.4	93.6	0.800
19	9	15	5.63	27.0	108.0	0.800
19	9	17.5	5.94	31.5	126.0	0.800
19	10	14	5.83	28.0	112.0	0.800
19	10	16	6.15	32.0	128.0	0.800
19	10	17.5	6.36	35.0	140.0	0.800
19	11	17.5	6.75	38.5	154.0	0.800
19	12	17.5	7.12	42.0	168.0	0.800
19	13	17.5	7.46	45.5	182.0	0.800
19	14	17.5	7.78	49.0	196.0	0.800
24	12	18	7.20	43.2	172.8	0.800
24	12	20	7.50	48.0	192.0	0.800
24	12	22.5	7.83	54.0	216.0	0.800
24	13	20	7.88	52.0	208.0	0.800
24	13	22.5	8.24	58.5	234.0	0.800
24	14	22.5	8.63	63.0	252.0	0.800
24	15	22.5	9.00	67.5	270.0	0.800
24	16	22.5	9.35	72.0	288.0	0.800
24	17	22.5	9.68	76.5	306.0	0.800
24	18	22.5	10.00	81.0	324.0	0.800

Note:

For integral bents, use a laminated bearing when taper over 1/8” is required.

751.11.2.3 Standard Pad Tables for Steel Girders

Standard Plain Fixed Bearing Pads for Steel Girders of Beams

L in.	W in.	S Single Layer	DL min kips	DLmax + LL kips	$\,\sigma _{TL}<ksi$
10	10	5.00	20.0	80.0	0.800
12	10	5.45	24.0	96.0	0.800
14	10	5.83	28.0	112.0	0.800
10	11	5.24	22.0	88.0	0.800
12	11	5.74	26.4	105.6	0.800
14	11	6.16	30.8	123.2	0.800
12	12	6.00	28.8	115.2	0.800
14	12	6.46	33.6	134.4	0.800
16	12	6.86	38.4	153.6	0.800
12	13	6.24	31.2	124.8	0.800
14	13	6.74	36.4	145.6	0.800
16	13	7.17	41.6	166.4	0.800
12	14	6.46	33.6	134.4	0.800
14	14	7.00	39.2	156.8	0.800
16	14	7.47	44.8	179.2	0.800
14	15	7.24	42.0	168.0	0.800
16	15	7.74	48.0	192.0	0.800
18	15	8.18	54.0	216.0	0.800
14	16	7.47	44.8	179.2	0.800
16	16	8.00	51.2	204.8	0.800
18	16	8.47	57.6	230.4	0.800
14	17	7.68	47.6	190.4	0.800
16	17	8.24	54.4	217.6	0.800
18	17	8.74	61.2	244.8	0.800
16	18	8.47	57.6	230.4	0.800
18	18	9.00	64.8	259.2	0.800
20	18	9.47	72.0	288.0	0.800
16	19	8.69	60.8	243.2	0.800
18	19	9.24	68.4	273.6	0.800
20	19	9.74	76.0	304.0	0.800
18	20	9.47	72.0	288.0	0.800
20	20	10.00	80.0	320.0	0.800
22	20	10.48	88.0	352.0	0.800

Use only plain pads at integral bents. Do not use sole plate.

Use girder chairs instead of widening beam.

Standard Laminated Bearing Pads for Steel Girders and Beams

B min.	L in.	W in.	S Int. Layer	S Ext. Layer	$\,h_{rt}$ max in.	$\,\triangle _{s}$ max in.	DL min kips	DLmax + LL kips	$\,\sigma _{TL}<ksi$
9	9	12	5.14	10.29	3.0	1.50	21.6	72.2	0.669
9	10	14	5.83	11.67	3.0	1.50	28.0	106.2	0.758
9	11	16	6.52	13.04	3.5	1.75	35.2	149.1	0.847
11	12	18	7.20	14.40	4.0	2.00	43.2	202.2	0.936
13	13	20	7.88	15.76	4.0	2.00	52.0	260.0	1.000
15	14	22	8.56	17.11	4.5	2.25	61.6	308.0	1.000
17	15	24	9.23	18.46	5.0	2.50	72.0	360.0	1.000
19	16	26	9.90	19.81	5.0	2.50	83.2	416.0	1.000
21	17	28	10.58	21.16	5.0	2.50	95.2	476.0	1.000
23	18	30	11.25	22.50	5.0	2.50	108.0	540.0	1.000
27	20	34	12.59	25.19	5.0	2.50	136.0	680.0	1.000
9	12	9	5.14	10.29	3.0	1.50	21.6	72.2	0.669
9	14	10	5.83	11.67	3.0	1.50	28.0	106.2	0.758
9	16	11	6.52	13.04	3.5	1.75	35.2	149.1	0.847
9	18	12	7.20	14.40	4.0	2.00	43.2	202.2	0.936
9	20	13	7.88	15.76	4.0	2.00	52.0	260.0	1.000
9	22	14	8.56	17.11	4.5	2.25	61.6	308.0	1.000
9	24	15	9.23	18.46	5.0	2.50	72.0	360.0	1.000
9	26	16	9.90	19.81	5.0	2.50	83.2	416.0	1.000
10	28	17	10.58	21.16	5.0	2.50	95.2	476.0	1.000
11	30	18	11.25	22.50	5.0	2.50	108.0	540.0	1.000
13	34	20	12.59	25.19	5.0	2.50	136.0	680.0	1.000

Where:

DL+LL max||= DC + DW + LL w/out dynamic load allowance

B min	= minimum allowable bottom flange width
$\,h_{rt}$ max	= maximum allowable total thickness of elastomer
$\,\triangle _{s}$ max	= maximum allowable shear deformation
DL min	= DC
DL max	= DC + DW

751.11.2.4 PTFE (Polytetraflouroethylene) Bearings

General

PTFE bearings shall be used if Δ_s > 2.5 in.

PTFE sliding bearing shall consist of a lower unit and an upper unit as follows:

The lower unit shall be PTFE bonded to a 1/8” stainless steel plate which is vulcanized to a laminated neoprene pad.

The upper unit shall be a 1/8” highly polished stainless steel plate welded to a steel sole plate.

70 Durometer hardness and shear modulus G = 0.2 ksi is to be used for sliding bearings.

Internal and external layers of elastomer shall be 0.5-inch and 0.25-inch thick, respectively.

The laminated neoprene pad shall contain at least one internal layer of elastomer. Providing an internal layer ensures that Method A may be used for design in accordance with LRFD 14.7.6.

The laminated neoprene elastomer pad shall meet the design requirements of 751.11.2.1, except shear deformation (i.e., deflection of bearing pad at first-slip) shall be checked as shown in the example below.

PTFE Sliding Surface Design

The following table represents the coefficients of friction for the design of PTFE sliding surfaces and are to be used instead of LRFD Table 14.7.2.5-1.

Design Coefficients of Friction at Various Vertical Pressures
PTFE Types	Coefficient of Friction, μ
PTFE Types	200 psi	400 psi	600 psi	800 psi	1000 psi
Unfilled - Flat	7%	7%	6%	6%	6%
Filled - Flat	20%	16%	13%	10%	8%
Unfilled - Dimpled	4%	3%	3%	3%	3%
Filled - Dimpled	5%	4%	4%	4%	4%

Note:
Vertical pressure = DLmax/A, where A is the total PTFE surface area minus the dimpled area, if applicable.
Intermediate values may be determined by interpolation. Integer values shall be used for plans reporting.
Dimpled PTFE sliding surfaces are the preferred PTFE type. Flat PTFE may be used with SPM or SLE approval. The contractor is allowed to use unfilled or filled pads so the filled values should be used in design.
Dimpled PTFE surfaces are lubricated.
Filled PTFE surfaces incorporate glass fibers, carbon fibers, or other chemically inert filler. The primary reason behind the application of such blended fibers is to reduce creep, i.e., cold flow, and wear.

PTFE Design Example – Filled -Dimpled

PTFE dimensions are the same as the bearing pad dimensions.

Service DLmax = 50 kips
Service LL = 73 kips
PTFE length, L = 11 in
PTFE width, W = 13 in
PTFE surface area, A = 143 - (30/100)143 = 100.1 in², assume dimpled area is 30% of total PTFE surface area
Total elastomer thickness, h_rt = 1.0 in
Elastomer shear modulus, G = 0.2 ksi

Check h_rt at first-slip:

DLmax /A = 50/100.1= 0.5 ksi = 500 psi
μ = 4%
Deflection of bearing pad at first-slip, Δ_s-slip = ɣ_FR(μ)DLmax(h_rt)/(A*G) = 1.0(4/100)50(1.0)/(100.1*0.2) = 0.1 in
h_rt ≥ 2Δ_s-slip = 2*0.1= 0.2 in OK
The PTFE sliding surface accommodates the remainder of the thermal movement at the bearing.

Check Average Contact Stress (LRFD 14.7.2.4):

This check will be met by satisfying the maximum compressive stress check for the elastomeric bearing pad.

DLmax/A ≤ 3.0 ksi
50/100.1= 0.5 ksi < 3.0 OK

(DLmax + LL)/A ≤ 4.5 ksi
(50+73)/100.1= 1.23 ksi < 4.5 OK

751.11.2.5 Multi-Rotational High Load Bearings

General

Pot and Disc bearings are the most commonly used multi-rotational bearings. Pot bearings carry vertical loads by compression of an elastomeric disc confined in a steel cylinder and accommodates rotation by deformation of the disc. Disc bearings accommodate vertical loads and rotation through the deformation of an unconfined or partially confined single elastomeric disc that is molded from a urethane compound.

Pot and disc bearings are commonly available in three different types:

1)Fixed – movement is not allowed and horizontal forces can be resisted from any direction.

2)Guided Expansion – allows for free movement in one direction. Horizontal forces can be resisted perpendicular to the free movement direction. For curved bridges, assume the free movement direction to be along a chord connecting the ends of the beam.

3)Expansion – unguided movement is allowed in all horizontal directions. Horizontal forces cannot be resisted.

The following design information shall be shown on the bearing sheets for each bearing location.

Type (fixed, guided-expansion, expansion)
Maximum Vertical Design Load
Minimum Vertical Design Load
Lateral Design Load (only when exceeding 10% of the Vertical Design Load)
Total Required Movement
Minimum Required Rotation
Slope of girder bottom
Bottom of girder elevations at centerline of bearing
Maximum Estimated Bearing Assembly Height
Location, Size, and Number of anchor bolts
Length and Width of masonry plate

Include the following note on the appropriate substructure sheets when multi-rotational bearings are used:

“Any modifications required to meet the height of bearings shown on bridge plans will be the responsibility of the contractor. Cost of any modification required shall be borne by the contractor.”

Inverted pot bearing configurations are not permitted.

Design

Pot or disc bearing design for structures shall follow LRFD 14.7.4 or 14.7.8 respectively.

Maximum and Minimum vertical loads shall be determined at the Service-I limit state. The minimum vertical load should not be less then 20 percent of the maximum vertical design load.

The lateral load provided by the manufacturer may be assumed 10 percent of the total vertical load. If applicable strength or extreme event load combinations result in a load greater than 10 percent, the required load shall be shown on the plans.

The total temperature movement required at a bent may be calculated using the procedure given in this section. Transverse movements should also be considered for large roadway widths. On wide roadways (greater than 40’) an increase in the standard clearance between the guide bar and bearing may be required.

The rotation due to factored loads, $\,\phi _{u}$ , shall be determined from the controlling strength limit state. The minimum rotation required shall include an additional 0.01 radians for fabrication and installation tolerances and uncertainties.

Due to a variety of preferences among multi-rotational bearing fabricators, explicit details are not provided in the plans. Instead, the fabricator determines the sizes of all the bearing components, from the masonry plate to the sole plate. As a guide, the following equation may be used to estimate the height of the assembly for design or the table below.

H = 6.5 + P/ 400

Where:

P = Total load calculated at the service-I limit state (excluding impact), k

H = Height of the bearing assembly measured from the bottom of the girder to the beam seat elevation, in

When determining the longitudinal load distributed to the substructure for expansion bearings, a friction coefficient of 0.05 shall be used.

Details

Bevel sole plate to match the slope of girder to the nearest 1/8” total difference in thickness across the plate.

When large movements are anticipated at a bearing location, three pairs of bearing stiffeners should be considered to help distribute the load.

Minimum of four anchor bolts are required for the connection of the masonry plate to the substructure.

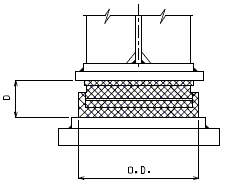
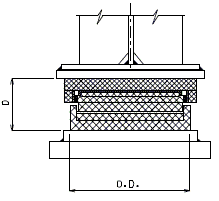
Estimated Pot Bearing Dimensions

The values shown are from one manufacturer and are based on assumed horizontal load requirements (Seismic Performance Category A). The designer should contact manufacturers with specific requirements for their products to get more detailed information on bearing dimensions.

These tables are to be used as a reference to assist the designer in estimating the size of the sole plate and the masonry plate.

Dimensions for Guided Expansion Bearing						Dimensions for Fixed
Total Vertical Load kips	Total Horiz. Load kips	A Inches	B Inches	O.D. Inches	D Inches	Total Vertical Load kips	Total Horiz. Load kips	A Inches	B Inches	O.D. Inches	D Inches
50	10	10	10.25	6.375	4.125	50	10	6.5	6.5	6.375	3
75	14.9	11	11.25	7.25	4.25	75	14.9	7.5	7.5	7.25	3
100	20	11.75	12.25	8.125	4.5	100	20	8.25	8.25	8.125	3.25
150	30	14.25	13.5	10	4.875	150	30	9.75	9.75	10	3.375
200	40	15.75	14.5	11.75	5	200	40	10.75	10.75	11.75	3.5
250	50	17.75	16	12.875	5.5	250	50	11.75	11.75	12.875	3.625
300	60	19.25	16.25	14.25	6	300	60	12.75	12.75	14.25	3.625
350	70	20	18.25	15.875	6.375	350	70	13.5	13.5	15.875	4.125
400	80	22.25	19	17.125	6.875	400	80	14.5	14.5	17.125	4.125
450	90	23	19	18.375	7	450	90	15	15	18.375	4.125
500	100	23.75	22.75	19.375	7	500	100	15.75	15.75	19.375	4.25
550	110	24.5	22.75	19.75	7.375	550	110	16.5	16.5	19.75	4.375
600	120	26.5	22.75	21.375	8.25	600	120	17	17	21.375	4.625
650	130	27.25	22.75	22.625	8.25	650	130	17.75	17.75	22.625	4.75
700	140	27.75	27.25	23.625	8.375	700	140	18.25	18.25	23.625	4.75
750	150	28.25	27.25	24.125	8.5	750	150	18.75	18.75	24.125	4.875
800	160	28.25	27.25	25	8.875	800	160	19.5	19.5	25	5
850	170	30.75	26.5	25.875	9.375	850	170	20	20	25.875	5
900	180	31.5	26.5	26.75	9.375	900	180	20.5	20.5	26.75	5
950	190	32	31.75	26.875	9.625	950	190	21	21	26.875	5.375
1000	200	32.25	31.75	27.5	9.75	1000	200	21.5	21.5	27.5	5.375
1100	220	33.5	31.75	28.875	9.875	1100	220	22.25	22.25	28.875	5.5
1200	240	26.75	26.5	30.75	10.375	1200	240	23.25	23.25	30.75	5.625
1300	260	27.875	27.5	32.25	10.625	1300	260	24	24	32.25	5.75
1400	280	28.75	28.25	32.625	11.125	1400	280	25	25	32.625	6.125
1500	300	29.625	29	33.5	11.5	1500	300	25.75	25.75	33.5	6.125
1600	320	30.5	29.75	34.75	11.75	1600	320	26.5	26.5	34.75	6.25
1700	340	31.5	30.5	35.75	12	1700	340	27.25	27.25	35.75	6.375
1800	360	32.5	31.5	37	12.25	1800	360	28	28	37	6.5
1900	380	33	33.125	37.375	12.5	1900	380	28.5	28.5	37.375	6.75
2000	400	33.75	35.125	38.125	12.5	2000	400	29.25	29.25	38.125	6.875
2100	420	34.25	38.125	39.125	12.375	2100	420	30	30	39.125	6.875
2200	440	35	38	40.125	12.625	2200	440	30.5	30.5	40.125	6.875
2300	460	35.875	38.75	41.625	13.125	2300	460	31.25	31.25	41.625	7.25
2400	480	36.25	41.25	41.875	13.125	2400	480	31.75	31.75	41.875	7.5
2500	500	37.125	42	42.625	13.375	2500	500	32.5	32.5	42.625	7.625
2600	520	37.875	42.75	43.5	13.625	2600	520	33	33	43.5	7.625
2700	540	38.5	43.25	44.375	13.75	2700	540	33.75	33.75	44.375	7.75
2800	560	39.125	43.75	45.25	14	2800	560	34.25	34.25	45.25	7.75
2900	580	40	44.5	46.25	14.125	2900	580	34.75	34.75	46.25	7.875
3000	600	40.5	45	46.625	14.375	3000	600	35.25	35.25	46.625	7.875
3100	620	41.125	45.5	45.625	14.75	3100	620	36	36	45.625	8.25
3200	640	41.75	46	46.875	15.125	3200	640	36.5	36.5	46.875	8.375
3300	660	42.375	46.5	47.625	15.375	3300	660	37	37	47.625	8.5
3400	680	43	47	48.5	15.5	3400	680	37.5	37.5	48.5	8.5
3500	700	43.75	47.75	49.375	15.625	3500	700	38	38	49.375	8.625

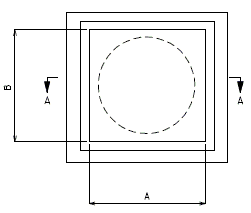
File:751.11 Fixed Bearing Plan.gif		File:751.11 Expansion Bearing Plan.gif
Fixed Bearing		Expansion Bearing
File:751.11 Fixed Bearing Elev.gif		File:751.11 Expansion Bearing Elev.gif
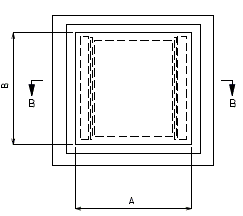
Section A-A		Section B-B
Fixed Bearing		Expansion Bearing

See table above for dimension A, B, O.D. and D.

751.11.3 Details

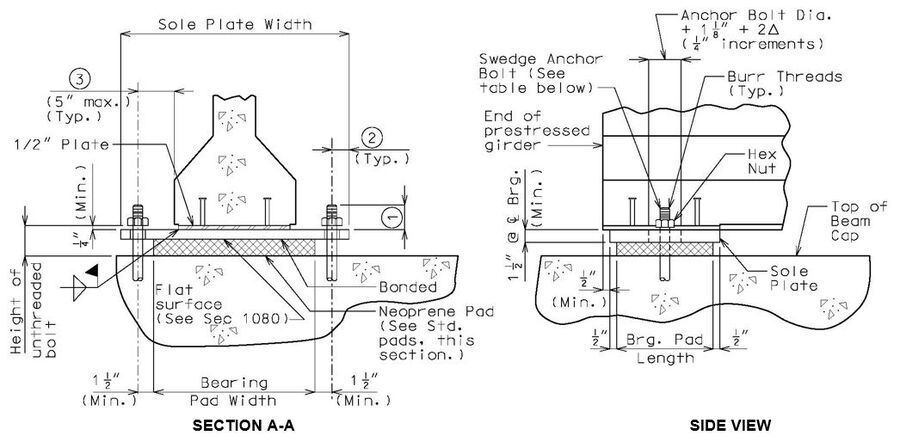
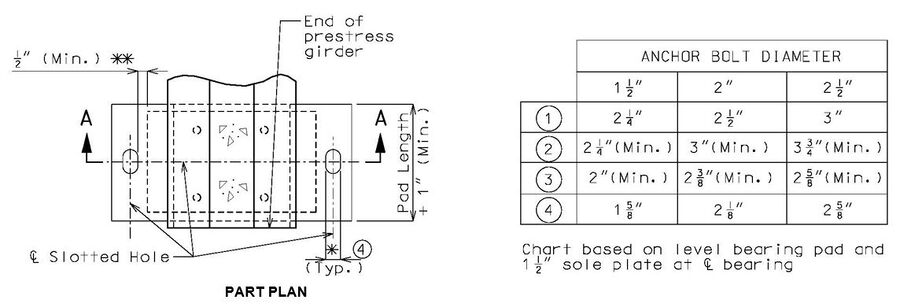
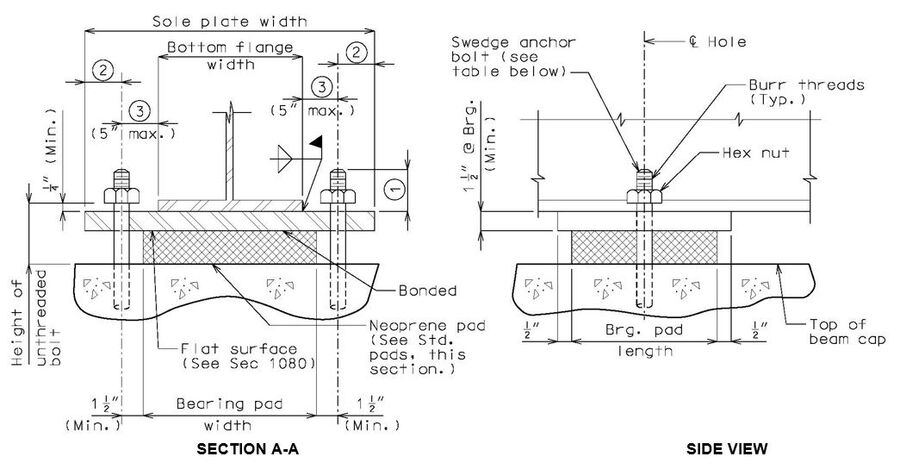
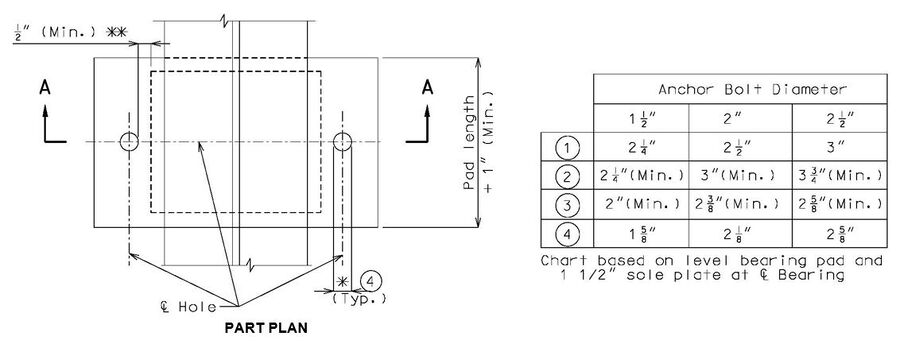
751.11.3.1 Elastomeric Bearings for P/S I-Girders

Expansion Bearings

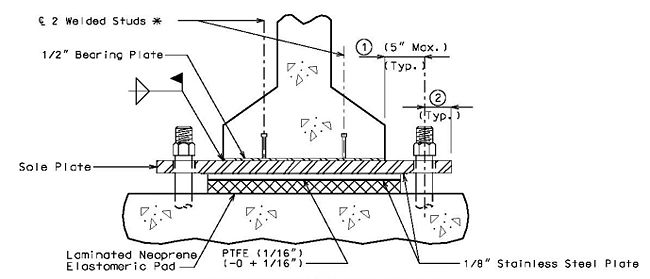
Note: The location of anchor bolts in relation to the slotted holes in the sole plate shall correspond with the temperature at the time of erection. At 60°F the slotted holes should center on the anchor bolts.

Notes:

For use of smaller or larger diameter anchor bolts than shown in the table “Anchor Bolt Diameter” and with approval of SPM or SLE, see Fabrication Operations Engineer.

Bevel sole plate to match the slope of girder to the nearest 1/8" total difference in thickness across the plate. Minimum thickness shall be 1 1/2" at centerline bearing and 1" at the edge of bearing. (By design)

For $\,\triangle$ > 2 1/2", use PTFE bearings.

* Slot width may be increased on wide roadways to accommodate lateral expansion. See Structural Project Manager.

** Designer should verify that minimum dimensions are met or neoprene will melt and flow into hole during manufacturing of bearings.

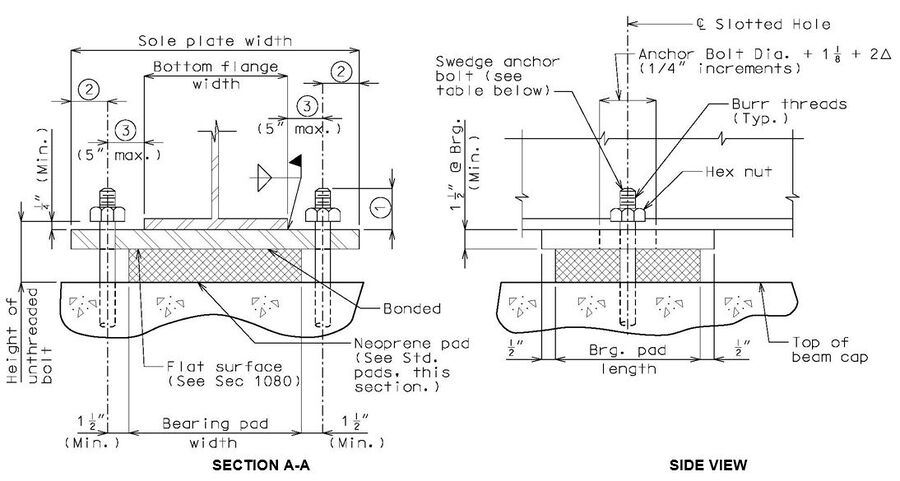
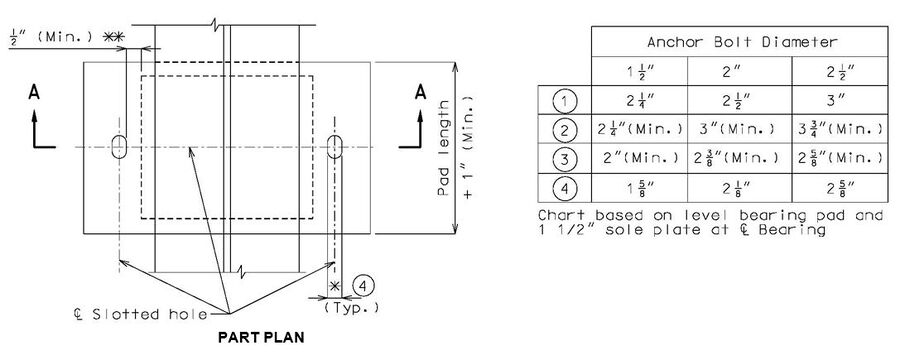
751.11.3.2 Elastomeric Bearings for Steel Girders

Laminated Expansion Bearings

Note: The location of anchor bolts in relation to the slotted holes in the sole plate shall correspond with the temperature at the time of erection. At 60°F the slotted holes should center on the anchor bolts.

Notes:

For use of smaller or larger diameter anchor bolts than shown in the table “Anchor Bolt Diameter” and with approval of SPM or SLE, see Fabrication Operations Engineer.

Bevel sole plate to match the slope of girder to the nearest 1/8" total difference in thickness across the plate. Minimum thickness shall be 1 1/2" at centerline bearing and 1" at the edge of bearing. (by design)

For $\,\triangle$ > 2 1/2", use PTFE bearings.

* Slot width may be increased on wide roadways to accommodate lateral expansion. See Structural Project Manager.

** Designer should verify that minimum dimensions are met or neoprene will melt and flow into hole during manufacturing of bearings.

Laminated Fixed Bearing

Notes:

For use of smaller or larger diameter anchor bolts than shown in the table “Anchor Bolt Diameter” and with approval of SPM or SLE, see Fabrication Operations Engineer.

Bevel sole plate to match the slope of girder to the nearest 1/8" total difference in thickness across the plate. Minimum thickness shall be 1 1/2" at centerline bearing and 1" at the edge of bearing. (by design)

* Slot width may be increased on wide roadways to accommodate lateral expansion. See Structural Project Manager.

** Design should verify that minimum dimensions are met or neoprene will melt and flow into hole during manufacturing of bearings.

Sole Plate Clipping Details
(for Steel Structures)

File:751.11 SolePlateClippingDetails Steel.gif SECTION THRU BEARING

Minimum edge distance shall be maintained on all holes in sole plate. Slotted holes shown are for expansion bearings.

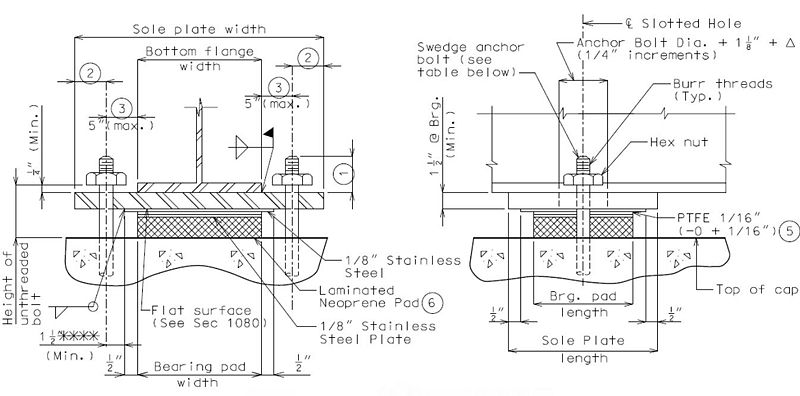
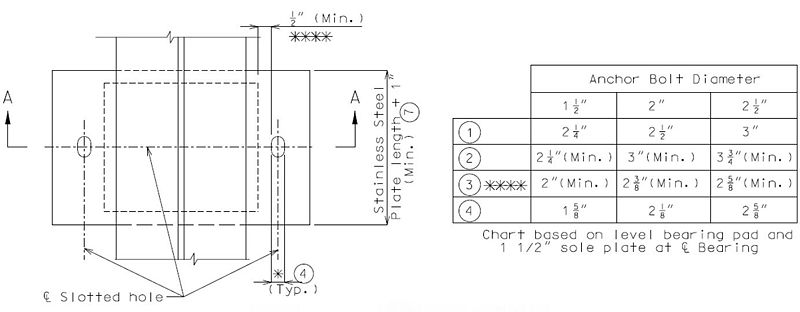
751.11.3.3 PTFE Bearings

Note: The location of anchor bolts in relation to the slotted holes in the sole plate shall correspond with the temperature at the time of erection. At 60°F the slotted holes should center on the anchor bolts.

SECTION A-A			SIDE VIEW


PART PLAN VIEW

File:751.11 PTFE Bearings Notes.gif

Notes:

For use of smaller or larger diameter anchor bolts than shown in the table “Anchor Bolt Diameter” and with approval of SPM or SLE, see Fabrication Operations Engineer.

* Slot width may be increased on wide roadways to accommodate lateral expansion. See Structural Project Manager.

**** Designer should verify that minimum dimensions are met or neoprene will melt and flow into the hole during manufacturing of bearing.

Either stopper plates, or both stopper plates and straps shall be used to restrain potential longitudinal, or longitudinal and transverse pad movement, respectively.

If anchor bolts are believed to be insufficient in restraining pad transverse movement, straps shall be used if the maximum distance between the anchor bolt and edge of girder is not exceeded. If straps are not required, or do not fit without exceeding this distance, stopper plates shall still be used and without recessed ends.

Details of the sole plate connection to the prestress girder.

SECTION THRU GIRDER

SECTION AT END OF GIRDER

* See EPG 751.22.3.4 Girder Reinforcement

751.11.3.4 Bearing Edge Distance

Steel or Prestress Girders

Beam Ledges

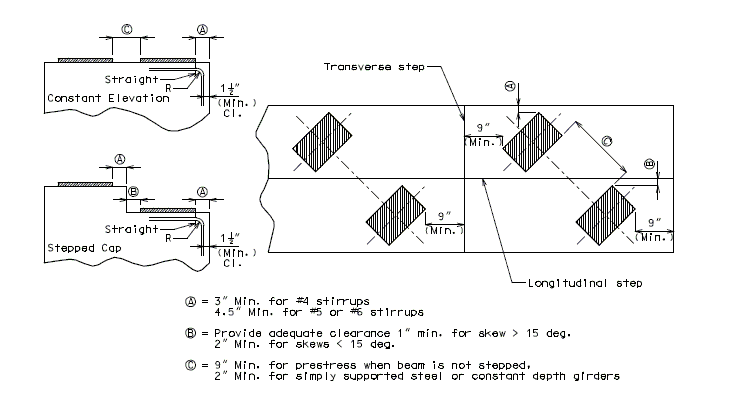
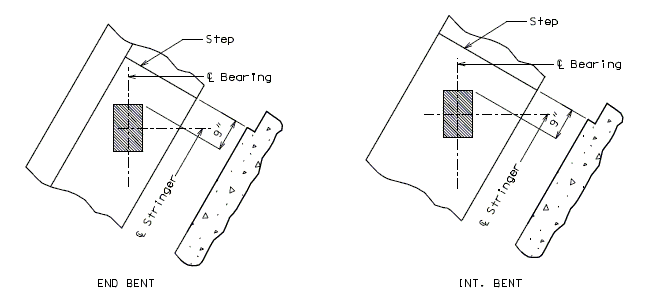
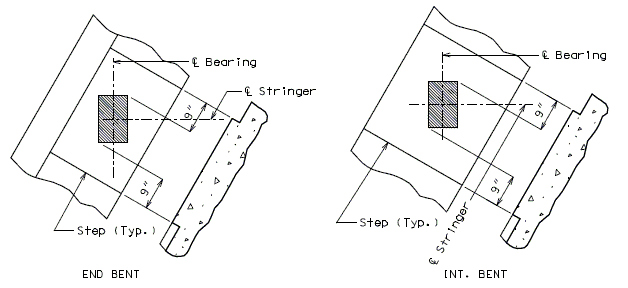
Heavy loads placed near the edge of a concrete surface can produce spalling. Two precautions should be taken to avoid the problem. Additional reinforcement should be placed under the load and around the corner. The bearing area of the load should not project beyond the straight portion of the stirrups, nor beyond the interior face of a transverse anchor bar (if one is provided). The recommended distance to a step, or the end of the bent cap in the transverse direction is 9 inches.

Provide minimum support length in accordance with Bridge Seismic Design Flowchart.

Bearing Clearance

When beams are placed to end at an intermediate bent, the clearance is governed by the bent up strands, diaphragm reinforcement, skew, and any vertical or horizontal curvature in the profile grade. A 9 inch minimum between bearing pads for prestressed girders is a general rule. For simply supported steel or constant depth girders the recommended minimum clearance is 2 inches between bearing pads. See Dimension "C". Also consider transverse girder offsets when using prestressed girders on horizontally curved alignments.

File:751.11 Bearing Edge Distance.gif

Check that all anchor bolts are located inside stirrup reinforcement.

Beam Steps

DIFFERENCE IN BEARING ELEV.	METHOD OF OBTAINING ELEVATION
1/8" or less	Retain in vertical dimension but use no fill plates Use the lower 1/8" for bent height.
1/4" thru 1/2"	Increase thickness of top plate of bearing or haunch.
5/8" thru 1" and up*	Monolithic bearing pad or step beam.

* Use same method of obtaining elevaton throughout a structure where practical. Do not mix monolithic bearing pads and steps.

File:751.11 Beam Steps- Step Beam.gif

STEP BEAM

File:751.11 Beam Steps- Monolithic Bearing Pads.gif

MONOLITHIC BEARING PADS

751.11.3.5 Anchor Bolts

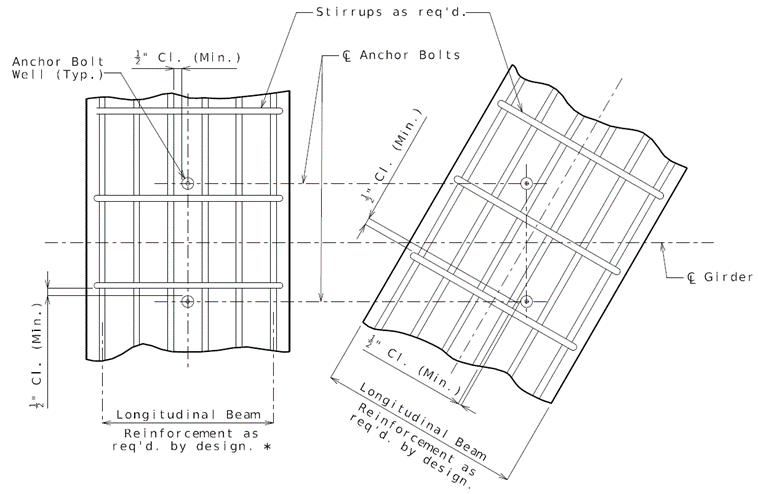
Show anchor bolt well locations and details on the plans.

Only removable forming material is required for forming anchor bolt wells in accordance with Sec 712 and as determined by the contractor which could include PVC, styrofoam, carboard, etc. In lieu of anchor bolts wells, anchor bolts holes may be drilled when permitted in the field as a contractor option, or where required which shall be noted on the plans.

Drilling anchor bolt holes should not be permitted in the field or made required on the plans unless reinforcement is detailed on plans to clear anchor bolt wells. An example of drilling needing to be required on the plans would be rehabilitations involving existing bent caps.

If uplift is a concern, determine capacity of anchorage based upon strength developed utilizing either well or drilled hole. Use engineering judgment in determining capacity of either system, i.e. friction capacity, grout capacity, anchor capacity. Identify specific method of anchoring required if there is a concern about differences in resistance capacity.

Clearance for reinforcement:

SQUARE		SKEWED

PART PLAN OF BEAM TOPS

(INT. BENTS SHOWN)

* Use even number of bars in beam tops for square bridges when possible.

Note: Details shown above are for information only. Place the following note on the plans, preferably with "General Notes."

"All reinforcing bars in the tops of substructure beams or caps shall be spaced to clear anchor bolt wells for bearings by at least 1/2"."

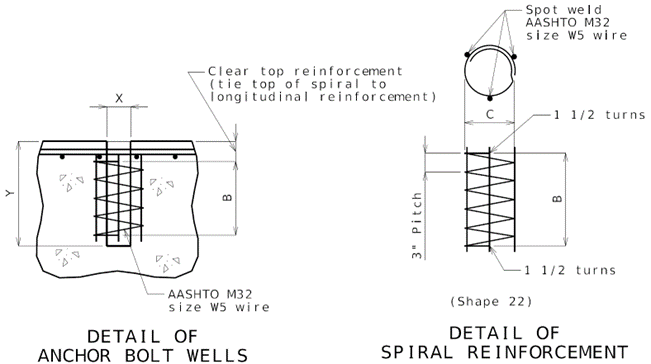
TABLE OF ANCHOR BOLT WELLS
Anchor Bolt Diameter	Well Diameter X	Well Depth Y	Spiral Depth B	Spiral Diameter C	W5 Nominal Length	W5 Actual Length	Bolt Extension into Concrete
1"	3"	12"	9"	8"	14'-10"	14-'6"	9"
1 1/4"	4"	15"	12"	9"	19’-7"	19'-1"	12"
1 1/2"	4"	18"	15"	9"	22'-8"	22'-2"	15"
2"	4"	21"	18"	9"	25'-9"	25'-2"	18"
2 1/2"	4"	28"	25"	9"	33'-1"	32'-4"	25"

Nominal length is based on out-to-out dimension. Actual length is the measured along the centerline of the W5 wire. Both nominal length and actual length includes the length of the three side wires.

Omit spiral on 21" WF if anchor bolt spacing is less than 6".

TABLE OF BOLT WEIGHTS
Description	1"	1 1/4"	1 1/2"	2"	2 1/2"
Unthreaded (#/in.)	----	----	0.5	0.89	1.39
Threaded (#/in.)	----	----	0.425	0.755	1.20
Nut (lbs.)	----	----	1.31	2.99	5.64
Weight used for entire bearing (#/ft.)	2.673	4.175	----	----	----

Epoxy coat anchor bolt wells at bents with expansion devices.

SWEDGE ANCHOR BOLT DETAILS


Bridge Standard Drawings
Bearings - BRG

751.11.3.6 Girder/Beam Chairs

General

Chairs shall be used at integral bents for prestressed and steel girders and beams if slope requirements cannot be met by elastomeric laminated fixed bearings. Chairs shall also be used for prestressed girders and beams at fixed intermediate bents where minimum dead load or slope requirements cannot be met by elastomeric laminated fixed bearings and under the smaller girder where girder sizes change.

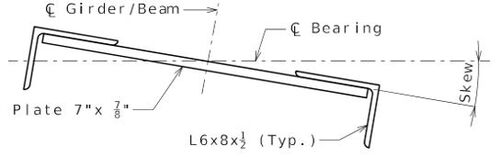
The following detail is not required on the plan sheets but illustrates the location of the bearing with respect to the 7-inch plate. See EPG 751.22.3.7.4 for how chairs are shown on the plan of beam.

PLAN OF CHAIR (Skewed bent)

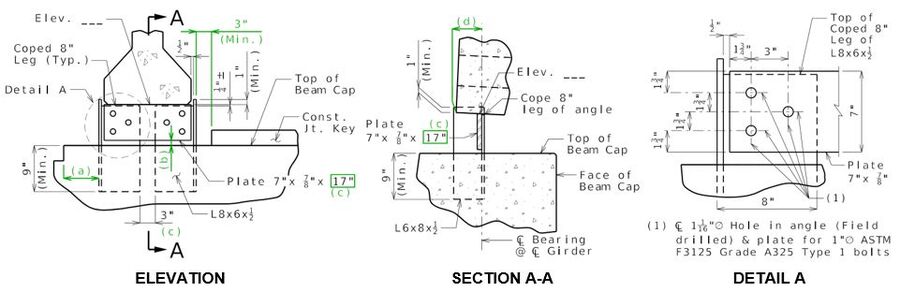
Chair Details

The following details shall be provided on the appropriate bent plan sheets. The beams steps and the construction key are shown to illustrate minimum distances, but these items are not required in these details on the plan sheets. Prestressed I girder shown, all other girders and beams are similar.

GIRDER CHAIR DETAILS

Detailing Guidance:
See EPG 751.50 (Notes A4a3.1 and G1.16 thru G1.19) for appropriate notes to be placed with details.
Green items are guidance and shall not be shown on plans.
	(a)	3" minimum to beam steps, 9" minimum to end of beam.
	(b)	1/2" minimum and 5" maximum. Use beam step if necessary. With a change in girder size, use thicker laminated pads on the tall girder side if necessary.
	(c)	These dimensions are based on a 17-inch bottom flange, revise accordingly for other widths.
	(d)	3" minimum for steel girders and beams.
		5" minimum for prestressed I girders and bulb-tee girders.
		3½" minimum for prestressed NU girders.

Maximum Service Limit State Load Reaction

The maximum service load reaction for the details shown shall be 90 kips.

This limit is based on ASTM A709 Grade 36 steel and bolt pattern shown with bolt threads in the shear plane.

Larger service load reactions will require modified details by design.

When determining service limit state load reaction use DC Loading (excluding barrier or railing) and an additional 50 lb/sf construction load.

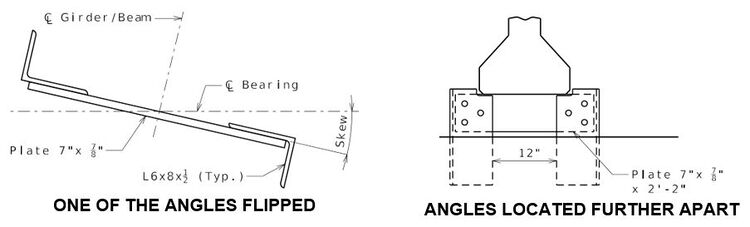
Possible Variations to Aid in Rebar Placement

The following variations of the above details shall be discussed with the Structural Project Manager or Structural Liaison Engineer if required to reduce conflict between the vertical angels and the beam reinforcement.

The gap and length of plate is variable and dependent on what is required for rebar placement. The details of the ends of closed concrete intermediate diaphragms will need to be modified from the details shown in EPG 751.22.3.7 Closed Concrete Intermediate Diaphragms.

751.11 Bearings

Contents

751.11.1 General

751.11.1.1 Bearing Types

751.11.1.2 Limit States and Load Factors

751.11.2 Design

751.11.2.1 Elastomeric Bearings

751.11.2.2 Standard Pad Tables for P/S I-Girders

751.11.2.3 Standard Pad Tables for Steel Girders

751.11.2.4 PTFE (Polytetraflouroethylene) Bearings

751.11.2.5 Multi-Rotational High Load Bearings

751.11.3 Details

751.11.3.1 Elastomeric Bearings for P/S I-Girders

751.11.3.2 Elastomeric Bearings for Steel Girders

751.11.3.3 PTFE Bearings

751.11.3.4 Bearing Edge Distance

751.11.3.5 Anchor Bolts

751.11.3.6 Girder/Beam Chairs

Navigation menu

Personal tools

Namespaces

Variants

Views

More

Search

navigation box|Navigation

Search Options

Contact Us

Other MoDOT Standards

Tools