Difference between revisions of "751.38 Spread Footings"
m |
m (Per BR, minor clarifications) |
||
| (6 intermediate revisions by the same user not shown) | |||
| Line 5: | Line 5: | ||
|} | |} | ||
| − | These guidelines address procedures for design of spread footings used as foundations for bridge piers, bridge abutments, retaining structures and other miscellaneous structures. | + | These guidelines address procedures for design of spread footings used as foundations for bridge piers, bridge abutments, retaining structures and other miscellaneous structures. The guidelines were established following load and resistance factor design (LRFD) concepts. The provisions provided herein are intended to produce foundations that achieve target reliabilities established by MoDOT for structures of different operational importance. The four classes of operational importance include minor or low volume route, major route, major bridge costing less than $100 million, and major bridge costing greater than $100 million. Additional background regarding development of these provisions and supportive information regarding use of these provisions is provided in the accompanying commentary. |
===751.38.1.1 Dimensions and Nomenclature=== | ===751.38.1.1 Dimensions and Nomenclature=== | ||
| Line 142: | Line 142: | ||
Resistance factors (<math>\boldsymbol \phi_b</math>) to be applied to the nominal resistance values (''q<sub>n</sub>'') determined according to the provisions of this article shall be established from Figure 751.38.3.1 based on the coefficient of variation of the mean uniaxial compressive strength, <math>COV_{\overline{q_u}}</math>. Values for ''q<sub>u</sub>'' and <math>COV_{\overline{q_u}}</math> shall be determined in accordance with methods described in [[321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation|EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation]] for the site and location in question. Values for design parameters ''q<sub>u</sub>'', ''m'' and ''s'' shall be taken as mean values for the rock between the base of the footing and a depth of ''B'' below the base of the footing. Values for <math>COV_{\overline{q_u}}</math> should similarly reflect the variability of the mean uniaxial compressive strength for the rock over the same depth range. | Resistance factors (<math>\boldsymbol \phi_b</math>) to be applied to the nominal resistance values (''q<sub>n</sub>'') determined according to the provisions of this article shall be established from Figure 751.38.3.1 based on the coefficient of variation of the mean uniaxial compressive strength, <math>COV_{\overline{q_u}}</math>. Values for ''q<sub>u</sub>'' and <math>COV_{\overline{q_u}}</math> shall be determined in accordance with methods described in [[321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation|EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation]] for the site and location in question. Values for design parameters ''q<sub>u</sub>'', ''m'' and ''s'' shall be taken as mean values for the rock between the base of the footing and a depth of ''B'' below the base of the footing. Values for <math>COV_{\overline{q_u}}</math> should similarly reflect the variability of the mean uniaxial compressive strength for the rock over the same depth range. | ||
| − | [[image:751.38.3.1.jpg|center|775px|thumb|<center>'''Fig. 751.38.3.1 Resistance factors for bearing resistance of spread footings on rock.'''</center>]] | + | [[image:751.38.3.1 2021.jpg|center|775px|thumb|<center>'''Fig. 751.38.3.1 Resistance factors for bearing resistance of spread footings on rock.'''</center>]] |
Values for ''C<sub>f1</sub>'' shall be taken from Table 751.38.3.1.1. Values for the rock mass parameters ''m'' and ''s'' can be established as: | Values for ''C<sub>f1</sub>'' shall be taken from Table 751.38.3.1.1. Values for the rock mass parameters ''m'' and ''s'' can be established as: | ||
| Line 220: | Line 220: | ||
Resistance factors (<math>\boldsymbol \phi_b</math>) to be applied to the nominal resistance values (''q<sub>n</sub>'') determined according to the provisions of this subarticle shall be established from Figure 751.38.3.2 based on the coefficient of variation of the mean uniaxial compressive strength <math>COV_{\overline{q_u}}</math>. Values for <math>\overline{q_u}</math> and <math>COV_{\overline{q_u}}</math> shall be determined in accordance with methods described in [[321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation|EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation]] for the site and location in question. Values for design parameter ''q<sub>u</sub>'' shall be taken as the mean value of the parameter for the rock between the base of the footing and a depth of ''B'' below the base of the footing. Values for <math>COV_{\overline{q_u}}</math> shall similarly reflect the variability of the mean uniaxial compressive strength over the same depth range. | Resistance factors (<math>\boldsymbol \phi_b</math>) to be applied to the nominal resistance values (''q<sub>n</sub>'') determined according to the provisions of this subarticle shall be established from Figure 751.38.3.2 based on the coefficient of variation of the mean uniaxial compressive strength <math>COV_{\overline{q_u}}</math>. Values for <math>\overline{q_u}</math> and <math>COV_{\overline{q_u}}</math> shall be determined in accordance with methods described in [[321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation|EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation]] for the site and location in question. Values for design parameter ''q<sub>u</sub>'' shall be taken as the mean value of the parameter for the rock between the base of the footing and a depth of ''B'' below the base of the footing. Values for <math>COV_{\overline{q_u}}</math> shall similarly reflect the variability of the mean uniaxial compressive strength over the same depth range. | ||
| − | [[image:751.38.3.2.jpg|center|thumb|700px|<center>'''Fig. 751.38.3.2 Resistance factors for bearing resistance for spread footings on weak rock.'''</center>]] | + | [[image:751.38.3.2 2021.jpg|center|thumb|700px|<center>'''Fig. 751.38.3.2 Resistance factors for bearing resistance for spread footings on weak rock.'''</center>]] |
The value of ''N<sub>c</sub>'' shall be taken as 5.0. The respective correction factors for footing shape and depth and for load inclination shall be computed as | The value of ''N<sub>c</sub>'' shall be taken as 5.0. The respective correction factors for footing shape and depth and for load inclination shall be computed as | ||
| Line 272: | Line 272: | ||
| − | [[image:table 751.38.3.3.jpg|center|775px|thumb|<center>'''Fig. 751.38.3.3 Resistance factors for bearing resistance for spread footings on cohesive soils.'''</center>]] | + | [[image:table 751.38.3.3 2021.jpg|center|775px|thumb|<center>'''Fig. 751.38.3.3 Resistance factors for bearing resistance for spread footings on cohesive soils.'''</center>]] |
===751.38.3.4 Bearing Resistance for Spread Footings on Cohesionless Soils=== | ===751.38.3.4 Bearing Resistance for Spread Footings on Cohesionless Soils=== | ||
| Line 290: | Line 290: | ||
Spread footings shall be dimensioned so that there is a small likelihood that footings will settle more than tolerable settlements, generally established from consideration of span length. This shall be accomplished by determining minimum footing dimensions for the appropriate site conditions in accordance with the content of this article. | Spread footings shall be dimensioned so that there is a small likelihood that footings will settle more than tolerable settlements, generally established from consideration of span length. This shall be accomplished by determining minimum footing dimensions for the appropriate site conditions in accordance with the content of this article. | ||
| − | Resistance factors provided in this | + | Resistance factors provided in this article were established to produce factored settlements that have a target probability of being exceeded. Target probabilities of exceedance were established by MoDOT for structures of different operational importance. Additional information regarding development of the resistance factors and application of the resistance factors for settlement calculations are provided in the commentary that accompanies these guidelines. |
| − | The method for determining minimum footing dimensions based on serviceability considerations shall be selected based on the material type present beneath the base of the footing. In general, EPG 751.38.4.1 shall be followed for footings founded in rock with uniaxial compressive strengths ( | + | The method for determining minimum footing dimensions based on serviceability considerations shall be selected based on the material type present beneath the base of the footing. In general, EPG 751.38.4.1 shall be followed for footings founded in rock with uniaxial compressive strengths (q<sub>u</sub>) greater than 100 ksf; EPG 751.38.4.2 shall be followed for footings founded in weaker rock with q<sub>u</sub> greater than 5 ksf but less than 100 ksf. The provisions in EPG 751.38.4.3 and EPG 751.38.4.4 shall be followed for footings founded in soil. |
===751.38.4.1 Settlement of Spread Footings on Rock (''q<sub>u</sub> ≥ 100 ksf'')=== | ===751.38.4.1 Settlement of Spread Footings on Rock (''q<sub>u</sub> ≥ 100 ksf'')=== | ||
| Line 335: | Line 335: | ||
For cases where the footing is underlain by practically homogeneous rock masses, ''H'' can be assumed to be equal to the footing dimension, ''B'' and values for <math>\overline{q_u}</math>, <math>COV_{\overline{q_u}}</math>, ''v'' and GSI shall be taken as the mean values of these parameters for the rock mass between the base of the footing and a depth of 2∙''H'' below the base of the footing. For cases where the rock beneath the footing is stratified, the value for ''H'' can be assumed to be the cumulative thickness of the more compressible strata within a depth of 2∙''B'' beneath the base of the footing. In such cases, values for <math>\overline{q_u}</math>, <math>COV_{\overline{q_u}}</math>, ''v'' and GSI shall be taken as the mean values of these parameters over the thickness of the more compressible strata. | For cases where the footing is underlain by practically homogeneous rock masses, ''H'' can be assumed to be equal to the footing dimension, ''B'' and values for <math>\overline{q_u}</math>, <math>COV_{\overline{q_u}}</math>, ''v'' and GSI shall be taken as the mean values of these parameters for the rock mass between the base of the footing and a depth of 2∙''H'' below the base of the footing. For cases where the rock beneath the footing is stratified, the value for ''H'' can be assumed to be the cumulative thickness of the more compressible strata within a depth of 2∙''B'' beneath the base of the footing. In such cases, values for <math>\overline{q_u}</math>, <math>COV_{\overline{q_u}}</math>, ''v'' and GSI shall be taken as the mean values of these parameters over the thickness of the more compressible strata. | ||
| − | [[image:751.38.4.1.jpg|center|700px|thumb|<center>'''Fig. 751.38.4.1 Resistance factors for settlement of spread footings on rock.'''</center>]] | + | [[image:751.38.4.1 2021.jpg|center|700px|thumb|<center>'''Fig. 751.38.4.1 Resistance factors for settlement of spread footings on rock.'''</center>]] |
====<center>''Table 751.38.4.1 Poisson’s Ratio values for intact rock (modified after Kulhawy, 1978''</center>==== | ====<center>''Table 751.38.4.1 Poisson’s Ratio values for intact rock (modified after Kulhawy, 1978''</center>==== | ||
| Line 406: | Line 406: | ||
Values for ''Φ<sub>S</sub>'' shall be established from Figure 751.38.4.2 based on the coefficient of variation of the mean uniaxial compressive strength, <math>COV_{\overline{q_u}}</math>, determined in accordance with methods described in [[321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation|EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation]] for the site and location in question. Values for ''v'' can be estimated from Table 751.38.4.1. | Values for ''Φ<sub>S</sub>'' shall be established from Figure 751.38.4.2 based on the coefficient of variation of the mean uniaxial compressive strength, <math>COV_{\overline{q_u}}</math>, determined in accordance with methods described in [[321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation|EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation]] for the site and location in question. Values for ''v'' can be estimated from Table 751.38.4.1. | ||
| − | [[image:751.38.4. | + | [[image:751.38.4.2 2021.jpg|center|700px||thumb|<Center>'''Fig. 751.38.4.2 Resistance factors for settlement of spread footings on weak rock.'''</center>]] |
| − | For cases where the footing is underlain by practically homogeneous rock masses, ''H'' can be assumed to be equal to the footing dimension, ''B'' and values for <math>\overline{q_u}</math>, <math> | + | For cases where the footing is underlain by practically homogeneous rock masses, ''H'' can be assumed to be equal to the footing dimension, ''B'' and values for <math>\overline{q_u}</math>, <math>COV_{\overline{q_u}}</math> and ''v'' shall be taken as the mean values of these parameters for the rock mass between the base of the footing and a depth of 2∙''H'' below the base of the footing. For cases where the rock beneath the footing is stratified, the value for ''H'' can be assumed to be the cumulative thickness of the more compressible strata within a depth of 2∙''B'' beneath the base of the footing. In such cases, values for <math>\overline{q_u}</math>, <math>COV_{\overline{q_u}}</math> and ''v'' shall be taken as the mean values of these parameters over the thickness of the more compressible strata. |
===751.38.4.3 Settlement of Spread Footings on Cohesive Soils=== | ===751.38.4.3 Settlement of Spread Footings on Cohesive Soils=== | ||
| Line 426: | Line 426: | ||
:''δ<sub>R</sub>'' = factored total settlement (consistent units of length) and | :''δ<sub>R</sub>'' = factored total settlement (consistent units of length) and | ||
| − | :''δ<sub>tol</sub>'' = tolerable settlement (consistent units of length) | + | :''δ<sub>tol</sub>'' = tolerable settlement (consistent units of length), |
the limit state is satisfied and the probability of footing settlement exceeding the tolerable settlement is less than or equal to the target probability established by MoDOT. If the factored total settlement is determined to exceed the tolerable settlement, the probability of footing settlement exceeding the tolerable value is greater than the target probability established by MoDOT. In such cases, the footing dimensions shall be increased until the factored total settlement is less than or equal to the tolerable settlement. | the limit state is satisfied and the probability of footing settlement exceeding the tolerable settlement is less than or equal to the target probability established by MoDOT. If the factored total settlement is determined to exceed the tolerable settlement, the probability of footing settlement exceeding the tolerable value is greater than the target probability established by MoDOT. In such cases, the footing dimensions shall be increased until the factored total settlement is less than or equal to the tolerable settlement. | ||
| − | Resistance factors provided in this | + | Resistance factors provided in this article were established to produce factored settlements that have a target probability of being exceeded. Target probabilities of exceedance were established by MoDOT for structures of different operational importance. Additional information regarding development of the resistance factors and application of the resistance factors for settlement calculations are provided in the commentary that accompanies these guidelines. |
'''Tolerable settlement''' | '''Tolerable settlement''' | ||
| Line 442: | Line 442: | ||
where: | where: | ||
| − | :''S'' = length of shortest bridge span adjacent to footing (consistent units of length) | + | :''S'' = length of shortest bridge span adjacent to footing (consistent units of length). |
'''Factored total settlement''' | '''Factored total settlement''' | ||
| Line 495: | Line 495: | ||
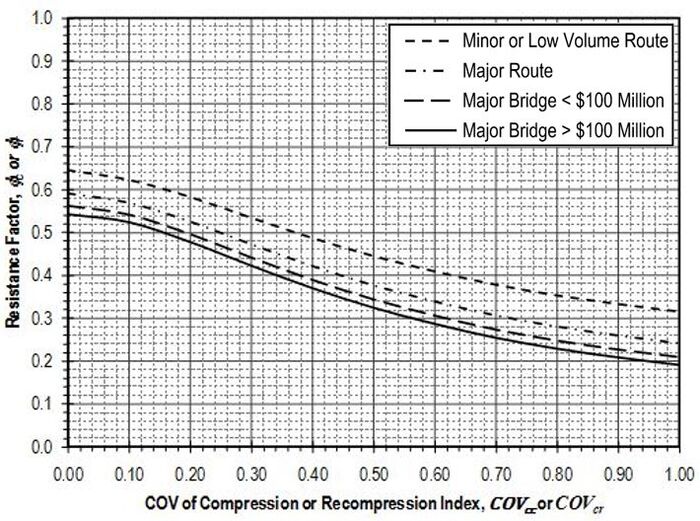
Values for ''Φ<sub>c</sub>'' and ''Φ<sub>r</sub>'' shall be established from Figure 751.38.4.3.1 based on the coefficient of variation of the mean compression index (<math>COV_{\overline{c_c}}</math>) and mean recompression index (<math>COV_{\overline{c_r}}</math>), respectively. Similarly, values for ''Φ<sub>p</sub>'' shall be established from Figure 751.38.4.3.2 based on the coefficient of variation of the mean maximum past vertical effective stress (<math>COV_{\overline{\sigma^'_p}}</math>). Coefficients of variation for each of these parameters shall be determined in accordance with methods described in [[321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation|EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation]]. | Values for ''Φ<sub>c</sub>'' and ''Φ<sub>r</sub>'' shall be established from Figure 751.38.4.3.1 based on the coefficient of variation of the mean compression index (<math>COV_{\overline{c_c}}</math>) and mean recompression index (<math>COV_{\overline{c_r}}</math>), respectively. Similarly, values for ''Φ<sub>p</sub>'' shall be established from Figure 751.38.4.3.2 based on the coefficient of variation of the mean maximum past vertical effective stress (<math>COV_{\overline{\sigma^'_p}}</math>). Coefficients of variation for each of these parameters shall be determined in accordance with methods described in [[321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation|EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation]]. | ||
| − | [[image:751.38.4. | + | [[image:751.38.4.3.1 2021.jpg|center|700px|thumb|<center>'''Fig. 751.38.4.3.1 Resistance factors for compression index and recompression index in calculation of settlement for spread footings on cohesive soils.'''</center>]] |
Where footings are underlain by compressible soils of substantial thickness, the soil beneath the footing shall be subdivided into several sublayers to account for potential changes in consolidation parameters and stress distribution beneath the footing. Compression of each of these sublayers shall be computed using Equation 751.38.4.5, 751.38.4.6 or 751.38.4.7, as appropriate, and the resulting values should be summed to arrive at the total settlement. For each sublayer, values for ''c<sub>c</sub>'', ''c<sub>r</sub>'' and ''e<sub>0</sub>'' shall be taken as the mean values of these parameters over the thickness of the sublayer. Values for ''H<sub>0</sub>'' shall be taken as the thickness of the respective sublayer. Values for ''σ'<sub>0</sub>'', ''σ'<sub>f</sub>'' and ''σ'<sub>p</sub>'' for each sublayer shall also be taken as the mean values over each sublayer, although this is often approximated by using values calculated for the center of the sublayer. Values used for <math>COV_{\overline{c_c}}</math> , <math>COV_{\overline{c_r}}</math> and <math>COV_{\overline{\sigma^'_p}}</math> shall be representative of the variability and uncertainty of the mean values for the respective parameters within each sublayer. | Where footings are underlain by compressible soils of substantial thickness, the soil beneath the footing shall be subdivided into several sublayers to account for potential changes in consolidation parameters and stress distribution beneath the footing. Compression of each of these sublayers shall be computed using Equation 751.38.4.5, 751.38.4.6 or 751.38.4.7, as appropriate, and the resulting values should be summed to arrive at the total settlement. For each sublayer, values for ''c<sub>c</sub>'', ''c<sub>r</sub>'' and ''e<sub>0</sub>'' shall be taken as the mean values of these parameters over the thickness of the sublayer. Values for ''H<sub>0</sub>'' shall be taken as the thickness of the respective sublayer. Values for ''σ'<sub>0</sub>'', ''σ'<sub>f</sub>'' and ''σ'<sub>p</sub>'' for each sublayer shall also be taken as the mean values over each sublayer, although this is often approximated by using values calculated for the center of the sublayer. Values used for <math>COV_{\overline{c_c}}</math> , <math>COV_{\overline{c_r}}</math> and <math>COV_{\overline{\sigma^'_p}}</math> shall be representative of the variability and uncertainty of the mean values for the respective parameters within each sublayer. | ||
| − | [[image:751.38.4. | + | [[image:751.38.4.3.2 2021.jpg|center|700px|thumb|<center>'''Fig. 751.38.4.3.2 Resistance factors for maximum past vertical effective stress used for calculation of settlement for spread footings on cohesive soils.'''</center>]] |
| Line 855: | Line 855: | ||
===751.38.8.3 Details=== | ===751.38.8.3 Details=== | ||
| − | ====751.38.8.3.1 Reinforcement==== | + | ====751.38.8.3.1 Spread Footing Reinforcement==== |
{|border="0" align="center" style="text-align:center" | {|border="0" align="center" style="text-align:center" | ||
| Line 888: | Line 888: | ||
These guidelines were developed from prior EPG guidelines with notable changes to the general approach for application of LRFD techniques as well as updated resistance factors based on probabilistic calibrations. Calibration analyses were performed following generally accepted procedures for calibration of resistance factors for geotechnical applications, but with modifications to permit several enhancements to be included in the guidelines. The most notable enhancements provided in the guidelines include: | These guidelines were developed from prior EPG guidelines with notable changes to the general approach for application of LRFD techniques as well as updated resistance factors based on probabilistic calibrations. Calibration analyses were performed following generally accepted procedures for calibration of resistance factors for geotechnical applications, but with modifications to permit several enhancements to be included in the guidelines. The most notable enhancements provided in the guidelines include: | ||
| − | :* | + | :* Use of resistance factors that are contingent upon the variability and uncertainty that exists in select design properties. |
| + | :* Adoption of different target reliability levels for foundations of structures of different operational importance. | ||
| − | |||
| − | Both of these enhancements are expected to produce efficient foundation designs while still maintaining appropriate safety and reliability for all classes of | + | Both of these enhancements are expected to produce efficient foundation designs while still maintaining appropriate safety and reliability for all classes of operational importance. Additional information regarding development of the methods provided in these guidelines can be found in Abu El-Ela et al. (2011) and Song et al. (2011). Additional information regarding target reliability values established for different classes of operational importance is provided in Bowders et al. (2011). |
| − | The | + | The four classes of operational importance include: |
| + | :* Minor and low volume route | ||
| + | :* Major route | ||
| + | :* Major bridge costing less than $100 million | ||
| + | :* Major bridge costing greater than $100 million. | ||
| − | + | These classifications are based on common MoDOT designations. The target reliability levels established for each limit state and operational importance were generally based upon consideration of highway bridges. However, the methods in this article can also be utilized for design of foundations for other structures including retaining walls and roadway signs. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | These classifications are based on common MoDOT designations. | ||
Calibration analyses performed to establish the resistance factors presented in this subarticle were performed using the latest knowledge of variability and uncertainty of applied loads (Kulicki et al., 2007), as well as using load factors that are currently in effect. The resistance factors provided in these guidelines are intended to produce foundations with reliabilities that are approximately equal to the target reliabilities established by MoDOT when utilized with current load factors. Since it is the combined effect of load and resistance factors that produce this reliability, the resistance factors provided are inherently coupled with current load factors and are contingent upon the uncertainty and variability in the applied loads that was presumed for the calibrations. As such, recalibration of resistance factors is required if alternative load factors are adopted, or if substantial revisions to current estimates of load variability and uncertainty are found. | Calibration analyses performed to establish the resistance factors presented in this subarticle were performed using the latest knowledge of variability and uncertainty of applied loads (Kulicki et al., 2007), as well as using load factors that are currently in effect. The resistance factors provided in these guidelines are intended to produce foundations with reliabilities that are approximately equal to the target reliabilities established by MoDOT when utilized with current load factors. Since it is the combined effect of load and resistance factors that produce this reliability, the resistance factors provided are inherently coupled with current load factors and are contingent upon the uncertainty and variability in the applied loads that was presumed for the calibrations. As such, recalibration of resistance factors is required if alternative load factors are adopted, or if substantial revisions to current estimates of load variability and uncertainty are found. | ||
| Line 1,022: | Line 1,018: | ||
This limiting value of angular distortion is based on theoretical consideration of the onset of deck cracking (Duncan and Tan, 1991). This limit is implicitly included in the methods provided in EPG 751.38.4.1 and EPG 751.38.4.2, while it is explicitly included in EPG 751.38.4.3. | This limiting value of angular distortion is based on theoretical consideration of the onset of deck cracking (Duncan and Tan, 1991). This limit is implicitly included in the methods provided in EPG 751.38.4.1 and EPG 751.38.4.2, while it is explicitly included in EPG 751.38.4.3. | ||
| − | The target probabilities of exceedance reflected in the resistance factors provided in EPG 751.38 correspond to the target values established by MoDOT based on economic considerations. | + | The target probabilities of exceedance reflected in the resistance factors provided in EPG 751.38 correspond to the target values established by MoDOT based on economic considerations. While use of alternative limits for tolerable settlement is possible, such use is not strictly appropriate since the target probabilities adopted by MoDOT for different classes of operational importance were established based on consequences associated with the limit provided in Equation Commentary 751.38.4.1. Other limits would generally require different target probabilities, and thus different resistance factors to achieve the same economic balance. |
As was the case in EPG 751.38.3, design procedures within this subarticle are categorized according to material type, including methods for design of spread footings founded upon “rock”, “weak rock”, “cohesive soil”, and “cohesionless soil”. While these categories serve to logically separate the guidelines according to design method, complexities present at some sites may lead to cases where multiple methods could potentially be used. In such cases, designers should utilize the method that is most appropriate for the conditions encountered, rather than selecting the method that produces the smallest or largest footing dimensions. | As was the case in EPG 751.38.3, design procedures within this subarticle are categorized according to material type, including methods for design of spread footings founded upon “rock”, “weak rock”, “cohesive soil”, and “cohesionless soil”. While these categories serve to logically separate the guidelines according to design method, complexities present at some sites may lead to cases where multiple methods could potentially be used. In such cases, designers should utilize the method that is most appropriate for the conditions encountered, rather than selecting the method that produces the smallest or largest footing dimensions. | ||
Latest revision as of 07:09, 29 June 2021
Contents
- 1 751.38.1 General
- 2 751.38.2 General Design Procedure and Limit States
- 3 751.38.3 Design for Axial Loading at Strength Limit States
- 3.1 751.38.3.1 Bearing Resistance for Spread Footings on Rock (qu ≥ 100 ksf)
- 3.2 751.38.3.2 Bearing Resistance for Spread Footings on Weak Rock (5 ksf ≤ qu ≤ 100 ksf)
- 3.3 751.38.3.3 Bearing Resistance for Spread Footings on Cohesive Soils (su ≤ 5,000 psf)
- 3.4 751.38.3.4 Bearing Resistance for Spread Footings on Cohesionless Soils
- 4 751.38.4 Design for Axial Loading at Serviceability Limit States
- 5 751.38.5 Modifications for Load Eccentricity
- 6 751.38.6 Design for Lateral Loading
- 7 751.38.7 Design for Overall Stability
- 8 751.38.8 Structural Design of Spread Footings
- 9 751.38.9 References
- 10 751.38.10 Commentary
- 10.1 Commentary on EPG 751.38.1 General
- 10.2 Commentary on EPG 751.38.1.2 General Design Considerations
- 10.3 Commentary on EPG 751.38.2 General Design Procedure and Limit States
- 10.4 Commentary on EPG 751.38.3 Design for Axial Loading at Strength Limit States
- 10.4.1 Commentary on EPG 751.38.3.1 Bearing Resistance for Spread Footings on Rock (qu ≥ 100 ksf)
- 10.4.2 Commentary on EPG 751.38.3.2 Bearing Resistance for Spread Footings on Weak Rock (5 ksf ≤ qu ≤ 100 ksf)
- 10.4.3 Commentary on EPG 751.38.3.3 Bearing Resistance for Spread Footings on Cohesive Soils (su ≤ 5,000 psf)
- 10.4.4 Commentary on EPG 751.38.3.4 Bearing Resistance for Spread Footings on Cohesionless Soils
- 10.5 Commentary on EPG 751.38.4 Design for Axial Loading at Serviceability Limit States
- 10.5.1 Commentary on EPG 751.38.4.1 Settlement of Spread Footings on Rock (qu ≥ 100 ksf)
- 10.5.2 Commentary on EPG 751.38.4.2 Settlement of Spread Footings on Weak Rock (5 ksf ≤ qu ≤ 100 ksf)
- 10.5.3 Commentary on EPG 751.38.4.3 Settlement of Spread Footings on Cohesive Soils
- 10.5.4 Commentary on EPG 751.38.4.4 Settlement of Spread Footings on Cohesionless Soils
- 10.6 Commentary on EPG 751.38.6 Design for Lateral Loading
- 10.7 Commentary on EPG 751.38.8 Structural Design of Spread Footings
- 10.8 Commentary on EPG 751.38.9 References
751.38.1 General
| Commentary for EPG 751.38.1 General |
These guidelines address procedures for design of spread footings used as foundations for bridge piers, bridge abutments, retaining structures and other miscellaneous structures. The guidelines were established following load and resistance factor design (LRFD) concepts. The provisions provided herein are intended to produce foundations that achieve target reliabilities established by MoDOT for structures of different operational importance. The four classes of operational importance include minor or low volume route, major route, major bridge costing less than $100 million, and major bridge costing greater than $100 million. Additional background regarding development of these provisions and supportive information regarding use of these provisions is provided in the accompanying commentary.
751.38.1.1 Dimensions and Nomenclature
Dimensions to be established in design include the bearing depth (depth to footing base) and the footing dimensions shown in Figure 751.38.1.1. Table 751.38.1.1 defines each dimension and provides relevant minimum and/or maximum values for the respective dimension.
Table 751.38.1.1 Summary of footing dimensions with minimum and maximum values
| Dimension | Description | Minimum Value | Maximum Value | Comment |
|---|---|---|---|---|
| D | Column diameter | 12” | -- | -- |
| B | Footing width | D+24” | -- | Min. 3” increments |
| L | Footing length | D+24”1 | -- | Min. 3” increments |
| A | Edge distance in width direction | 12” | -- | -- |
| A’ | Edge distance in length direction | 12” | -- | -- |
| t | Footing thickness | 30” or D2 | 72” | Min. 3” increments |
| 1 Minimum of 1/6 x distance from top of beam to bottom of footing | ||||
| 2 For column diameters ≥ 48”, use minimum value of 48” | ||||
The nomenclature used in these guidelines has intentionally been selected to be consistent with that used in the AASHTO LRFD Bridge Design Specifications (AASHTO, 2009) to the extent possible to avoid potential confusion with methods provided in those specifications. By convention, references to other provisions of the MoDOT Engineering Policy Guide are indicated as “EPG XXX.XX” throughout these guidelines where the Xs are replaced with the appropriate article numbers. Similarly, references to provisions within the AASHTO LRFD Bridge Design Specifications are indicated as “LRFD XXX.XX”.
751.38.1.2 General Design Considerations
| Commentary for EPG 751.38.1.2 General Design Considerations |
Footings shall be founded to bear a minimum of 36 in. below the finished elevation of the ground surface. In cases where scour, erosion, or undermining can be reasonably anticipated, footings shall bear a minimum of 36 in. below the maximum anticipated depth of scour, erosion, or undermining.
Footing size shall be proportioned so that stresses under the footing are as uniform as practical at the service limit state.
Long, narrow footings supporting individual columns should be avoided unless space constraints or eccentric loading dictate otherwise, especially on foundation material of low capacity. In general, spread footings should be made as close to square as possible. The length to width ratio of footings supporting individual columns should not exceed 2.0, except on structures where the ratio of longitudinal to transverse loads or site constraints makes use of such a limit impractical.
Footings located near to rock slopes (e.g. rock cuts, river bluffs, etc.) shall be located so that the footing is founded beyond a prohibited region established by a line inclined from the horizontal passing through the toe of the slope as shown in Figure 751.38.1.2. The boundary of the prohibited region shall be established by the Geotechnical Section. For the purposes of this provision, the toe of the slope shall be the point on the slope that produces the most severe location for the active zone. Exceptions to this provision shall only be made with specific approval of the Geotechnical Section and shall only be granted if overall stability can be demonstrated as provided in EPG 751.38.7.
Footings located near to soil slopes shall be evaluated for overall stability as provided in EPG 751.38.7 unless they are located a minimum distance of 2B beyond the crest of the slope.
751.38.1.3 Related Provisions
The provisions in these guidelines were developed presuming that design parameters required to apply the provisions are established following current MoDOT site characterization protocols as described in EPG 321. Specific attention is drawn to EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation. The provisions provided in this subarticle presume that parameter variability, as generally represented by the coefficient of variation (COV), is established following procedures in EPG 321.3.
751.38.2 General Design Procedure and Limit States
| Commentary for EPG 751.38.2 General Design Procedure and Limit States |
Spread footings shall be dimensioned to safely support the anticipated design loads without excessive deflections. Footing dimensions shall be established based on project specific requirements, site constraints, and the requirements of this subarticle. Footings shall be sized at the applicable strength and serviceability limit states according to EPG 751.38.3 and EPG 751.38.4; the greatest minimum dimensions established from consideration of each of these limit states shall govern the final design dimensions as long as they exceed the minimum dimensions specified in EPG 751.38.1. Final design dimensions shall also be increased for cases with significant load eccentricity in accordance with EPG 751.38.5.
At a minimum, footings shall be designed to satisfy the Strength I and Service I limit states.
751.38.3 Design for Axial Loading at Strength Limit States
| Commentary for EPG 751.38.3 Design for Axial Loading at Strength Limit States |
In general, spread footings shall be sized for strength limit states so that the factored bearing resistance exceeds the factored loads for the strength limit state of interest. This shall be accomplished by determining the minimum footing dimensions, B and L, so that the following condition is satisfied
| (consistent units of area) | Equation 751.38.3.1.1 |
where:
- B = minimum footing width (consistent units of length),
- L = minimum footing length (consistent units of length),
- = factored load for the appropriate strength limit state (consistent units of force) and
- qR = factored bearing resistance (consistent units of stress).
The factored bearing resistance shall be established as
| (consistent units of area) | Equation 751.38.3.2 |
where:
- qR = factored bearing resistance (consistent units of stress),
- Φb = resistance factor for bearing resistance determined in accordance with this article (dimensionless) and
- qn = nominal bearing resistance determined in accordance with this article (consistent units of stress).
For cases with eccentric loading, the modified footing dimensions, B’ and L’, shall be used for evaluations at strength limit states instead of the actual footing dimensions:
| (consistent units of area) | Equation 751.38.3.1.2 |
where B’ and L’ are established as stipulated in EPG 751.38.5:
- B' = modified footing width to account for load eccentricity (consistent units of length) and
- L' = modified footing length to account for load eccentricity (consistent units of length).
Final minimum footing dimensions shall not be less than those stipulated in EPG 751.38.1.
The method for determining the factored bearing resistance shall be selected based on the material type present beneath the base of the footing. In general, EPG 751.38.3.1 shall be followed for footings founded on rock with uniaxial compressive strengths (qu) greater than 100 ksf; EPG 751.38.3.2 shall be followed for footings founded on weak rock with qu greater than 5 ksf but less than 100 ksf. The provisions in EPG 751.38.3.3 and EPG 751.38.3.4 shall be followed for footings founded on soil.
751.38.3.1 Bearing Resistance for Spread Footings on Rock (qu ≥ 100 ksf)
| Commentary for EPG 751.38.3.1 Bearing Resistance for Spread Footings on Rock (qu ≥ 100 ksf) |
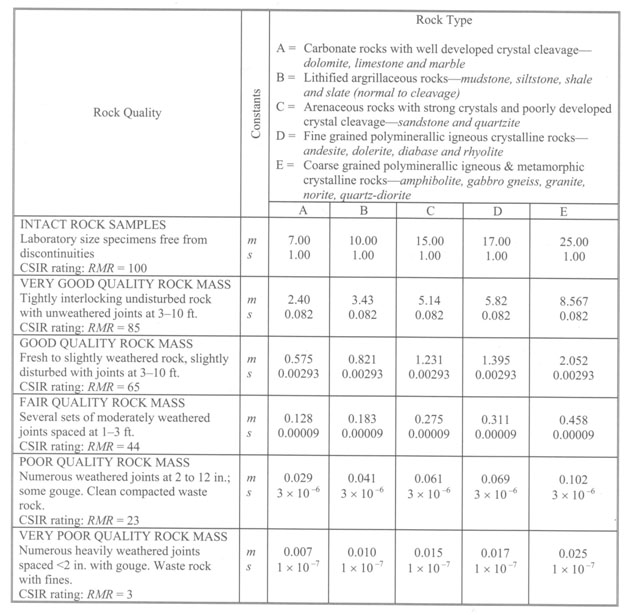
The nominal bearing resistance for spread footings on rock shall be calculated as a function of the mean uniaxial compressive strength of the intact rock according to (adapted from Wyllie, 1999):
| (consistent units of stress) | Equation 751.38.3.3 |
where:
- m and s = empirical constants describing the rock mass strength (dimensionless),
- Cf1 = correction factor to account for footing shape (dimensionless) and
- = mean value of the uniaxial compressive strength of intact rock core (consistent units of stress).
Resistance factors () to be applied to the nominal resistance values (qn) determined according to the provisions of this article shall be established from Figure 751.38.3.1 based on the coefficient of variation of the mean uniaxial compressive strength, . Values for qu and shall be determined in accordance with methods described in EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation for the site and location in question. Values for design parameters qu, m and s shall be taken as mean values for the rock between the base of the footing and a depth of B below the base of the footing. Values for should similarly reflect the variability of the mean uniaxial compressive strength for the rock over the same depth range.
Values for Cf1 shall be taken from Table 751.38.3.1.1. Values for the rock mass parameters m and s can be established as:
| (dimensionless) | Equation 751.38.3.4 | |
| (dimensionless) | Equation 751.38.3.5a | |
| (dimensionless) | Equation 751.38.3.5b |
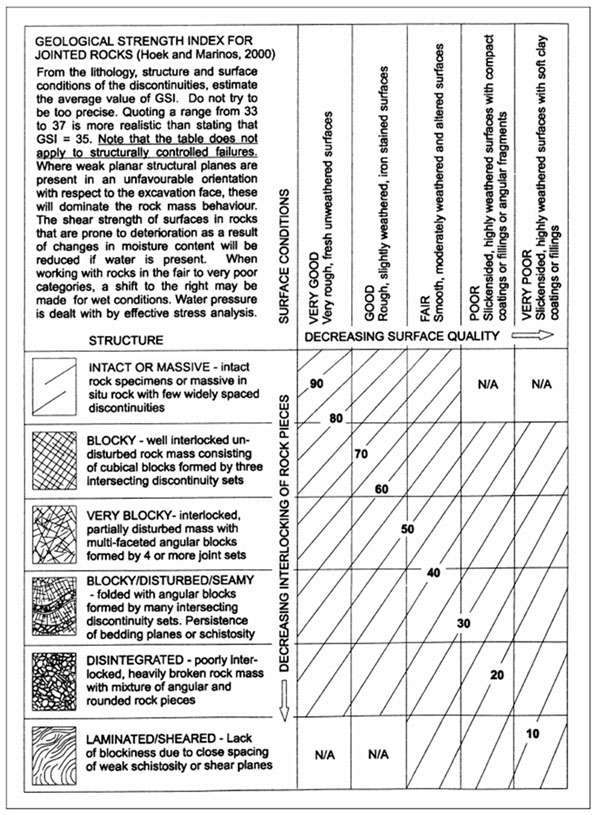
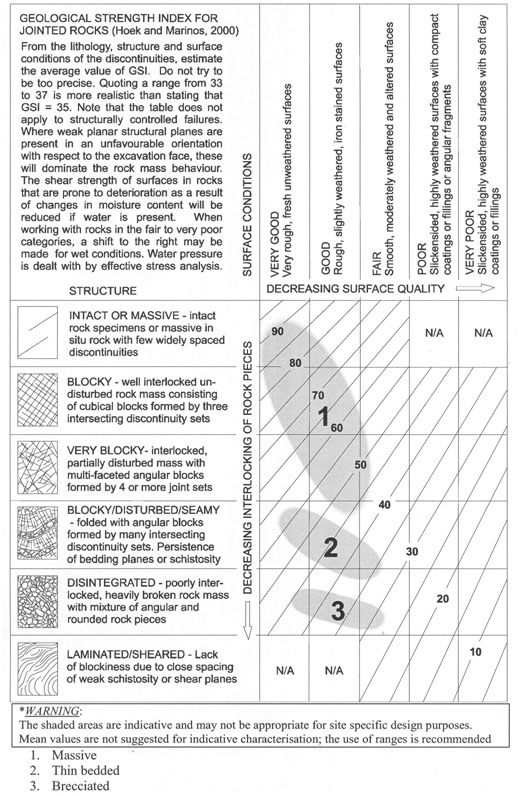
where mi is a material constant corresponding to rock type and GSI is the Geological Strength Index. The value for mi can be estimated from Table 751.38.3.1.2 or determined more precisely from triaxial tests (Hoek and Brown, 1997). For routine design, mi can be approximated as 10 for limestones and dolomites, as 6 for shales, siltstones, and mudstones and as 17 for sandstones. Values for GSI can be estimated from rock mass characterizations using the Rock Mass Rating (RMR) system for rock masses with RMR greater than 25 (Hoek and Brown, 1997). Using this approach, GSI is calculated as:
| (dimensionless) | Equation 751.38.3.6 |
where
Ri = Rock Mass Rating system rating parameters (dimensionless). GSI is thus equivalent to the RMR value with the groundwater rating term, R5, taken as 10.
Values for GSI to be used in Equations 751.38.3.4 and 751.38.3.5, or values for m and s to be used in Equation 751.38.3.3, can also be established using alternative methods described in Commentary for EPG 751.38.3.1.
The nominal bearing resistance predicted using Equation 751.38.3.3 shall be limited to a maximum value of 200 ksf unless greater bearing resistance can be verified by a load test.
Table 751.38.3.1.1 Correction factors to account for footing shape for evaluation of bearing resistance for spread footings on rock (from Wyllie, 1999)
| Footing Shape | Cf1 | |
|---|---|---|
| Strip (L/B > 6) | 1.00 | |
| Rectangular | L/B = 2 | 1.12 |
| L/B = 5 | 1.05 | |
| Square (L/B = 1) | 1.25 | |
| Circular (L/B = 1) | 1.20 | |

** These values are for intact rock specimens tested normal to bedding or foliation. The value of mi will be significantly different if failure occurs along a weakness plane.
751.38.3.2 Bearing Resistance for Spread Footings on Weak Rock (5 ksf ≤ qu ≤ 100 ksf)
| Commentary for EPG 751.38.3.2 Bearing Resistance for Spread Footings on Weak Rock (5 ksf ≤ qu ≤ 100 ksf) |
The nominal bearing resistance for spread footings on weak rock (e.g. mudstone, siltstone, weak sandstone, etc.) shall be calculated as a function of the mean uniaxial compressive strength of the rock according to (adapted from Wyllie, 1999):
| (consistent units of stress) | Equation 751.38.3.7 |
where:
- = mean value of the uniaxial compressive strength of the rock (consistent units of stress),
- Nc = bearing capacity factor (dimensionless),
- sc = correction factor to account for footing shape (dimensionless),
- dc = correction factor to account for footing depth (dimensionless) and
- ic = correction factor to account for inclination of the factored load (dimensionless).
Resistance factors () to be applied to the nominal resistance values (qn) determined according to the provisions of this subarticle shall be established from Figure 751.38.3.2 based on the coefficient of variation of the mean uniaxial compressive strength . Values for and shall be determined in accordance with methods described in EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation for the site and location in question. Values for design parameter qu shall be taken as the mean value of the parameter for the rock between the base of the footing and a depth of B below the base of the footing. Values for shall similarly reflect the variability of the mean uniaxial compressive strength over the same depth range.
The value of Nc shall be taken as 5.0. The respective correction factors for footing shape and depth and for load inclination shall be computed as
| (dimensionless) | Equation 751.38.3.8 | |
| (dimensionless) | Equation 751.38.3.9 | |
| (dimensionless) | Equation 751.38.3.10 |
where:
- B and L = footing width and length, respectively (consistent units of length),
- θ = inclination of the factored resultant column load measured from the vertical (degrees) and
- Df = depth of embedment of footing.
The nominal bearing resistance predicted using Equation 751.38.3.7 shall be limited to a maximum value of 200 ksf unless greater bearing resistance can be verified by a load test.
751.38.3.3 Bearing Resistance for Spread Footings on Cohesive Soils (su ≤ 5,000 psf)
| Commentary for EPG 751.38.3.3 Bearing Resistance for Spread Footings on Cohesive Soils (su ≤ 5,000 psf) |
The nominal bearing resistance for spread footings on cohesive soils shall be calculated as a function of the mean undrained shear strength of the soil according to:
| (consistent units of stress) | Equation 751.38.3.11 |
where:
- = mean value of the undrained shear strength of the soil (consistent units of stress),
- Nc = bearing capacity factor (dimensionless),
- sc = correction factor to account for footing shape (dimensionless),
- dc = correction factor to account for footing depth (dimensionless) and
- ic = correction factor to account for inclination of the factored load (dimensionless).
Resistance factors () to be applied to the nominal resistance values (qn) determined according to the provisions of this subarticle shall be established from Figure 751.38.3.3 based on the coefficient of variation of the mean undrained shear strength, . Values for and shall be determined in accordance with methods described in EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation for the site and location in question. Values for design parameter shall be taken as the mean value for the soil between the base of the footing and a depth of B below the base of the footing. Values for shall similarly reflect the variability of the mean soil shear strength over the same range of depths.
The value of Nc shall be taken as 5.0. The respective correction factors shall be computed using Equations 751.38.3.8, 751.38.3.9 and 751.38.3.10.
751.38.3.4 Bearing Resistance for Spread Footings on Cohesionless Soils
| Commentary for EPG 751.38.3.4 Bearing Resistance for Spread Footings on Cohesionless Soils |
Spread footings on cohesionless soils shall be designed according to applicable sections of the current AASHTO LRFD Bridge Design Specifications.
751.38.4 Design for Axial Loading at Serviceability Limit States
| Commentary for EPG 751.38.4 Design for Axial Loading at Serviceability Limit States |
Spread footings shall be dimensioned so that there is a small likelihood that footings will settle more than tolerable settlements, generally established from consideration of span length. This shall be accomplished by determining minimum footing dimensions for the appropriate site conditions in accordance with the content of this article.
Resistance factors provided in this article were established to produce factored settlements that have a target probability of being exceeded. Target probabilities of exceedance were established by MoDOT for structures of different operational importance. Additional information regarding development of the resistance factors and application of the resistance factors for settlement calculations are provided in the commentary that accompanies these guidelines.
The method for determining minimum footing dimensions based on serviceability considerations shall be selected based on the material type present beneath the base of the footing. In general, EPG 751.38.4.1 shall be followed for footings founded in rock with uniaxial compressive strengths (qu) greater than 100 ksf; EPG 751.38.4.2 shall be followed for footings founded in weaker rock with qu greater than 5 ksf but less than 100 ksf. The provisions in EPG 751.38.4.3 and EPG 751.38.4.4 shall be followed for footings founded in soil.
751.38.4.1 Settlement of Spread Footings on Rock (qu ≥ 100 ksf)
| Commentary for EPG 751.38.4.1 Settlement of Spread Footings on Rock (qu ≥ 100 ksf) |
For spread footings on rock, the minimum footing dimensions shall be established from the following:
| (ft2) | Equation 751.38.4.1 |
where :
- B = minimum footing width (feet),
- L = minimum footing length (feet),
- = factored load for the appropriate serviceability limit state (kips)
- v = mean value of Poisson’s ratio (dimensionless),
- = mean value for the uniaxial compressive strength (ksf),
- GSI = mean value for the geological strength index (dimensionless),
- H = thickness of rock subjected to stress below the footing (feet),
- S = minimum span length for spans adjacent to the footing (feet) and
- ΦS = resistance factor for settlement of spread footings on rock (dimensionless).
Note that this expression is dimensional so values must be entered in the units specified.
Values for ΦS shall be established from Figure 751.38.4.1 based on the coefficient of variation of the mean uniaxial compressive strength, , determined in accordance with methods described in EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation for the site and location in question. Values for v can be estimated from Table 751.38.4.1. Values for GSI can be estimated using methods outlined in EPG 751.38.3.1, or using alternative methods described in the commentary to that subarticle.
For cases where the footing is underlain by practically homogeneous rock masses, H can be assumed to be equal to the footing dimension, B and values for , , v and GSI shall be taken as the mean values of these parameters for the rock mass between the base of the footing and a depth of 2∙H below the base of the footing. For cases where the rock beneath the footing is stratified, the value for H can be assumed to be the cumulative thickness of the more compressible strata within a depth of 2∙B beneath the base of the footing. In such cases, values for , , v and GSI shall be taken as the mean values of these parameters over the thickness of the more compressible strata.
Table 751.38.4.1 Poisson’s Ratio values for intact rock (modified after Kulhawy, 1978
| Rock Type | # Values | # Rock Types | Poisson’s Ratio, v | Standard Deviation | ||
|---|---|---|---|---|---|---|
| Maximum | Minimum | Mean | ||||
| Granite | 22 | 22 | 0.39 | 0.09 | 0.20 | 0.08 |
| Gabbro | 3 | 3 | 0.20 | 0.16 | 0.18 | 0.02 |
| Diabase | 6 | 6 | 0.38 | 0.20 | 0.29 | 0.06 |
| Basalt | 11 | 11 | 0.32 | 0.16 | 0.23 | 0.05 |
| Quartzite | 6 | 6 | 0.22 | 0.08 | 0.14 | 0.05 |
| Marble | 5 | 5 | 0.40 | 0.17 | 0.28 | 0.08 |
| Gneiss | 11 | 11 | 0.40 | 0.09 | 0.22 | 0.09 |
| Schist | 12 | 11 | 0.31 | 0.02 | 0.12 | 0.08 |
| Sandstone | 12 | 9 | 0.46 | 0.08 | 0.20 | 0.11 |
| Siltstone | 3 | 3 | 0.23 | 0.09 | 0.18 | 0.06 |
| Shale | 3 | 3 | 0.18 | 0.03 | 0.09 | 0.06 |
| Limestone | 19 | 19 | 0.33 | 0.12 | 0.23 | 0.06 |
| Dolostone | 5 | 5 | 0.35 | 0.14 | 0.29 | 0.08 |
751.38.4.2 Settlement of Spread Footings on Weak Rock (5 ksf ≤ qu ≤ 100 ksf)
| Commentary for EPG 751.38.4.2 Settlement of Spread Footings on Weak Rock (5 ksf ≤ qu ≤ 100 ksf) |
Spread footings founded on weak rock shall have the following minimum dimensions:
| (ft2) | Equation 751.38.4.2 |
where:
- B = minimum footing width (feet),
- L = minimum footing length (feet),
- = factored load for the appropriate serviceability limit state (kips)
- v = mean value of Poisson’s ratio (dimensionless),
- = mean value for the uniaxial compressive strength (ksf),
- H = thickness of rock subjected to stress below the footing (feet),
- S = minimum span length for spans adjacent to the footing (feet) and
- ΦS = resistance factor for settlement of spread footings on weak rock (dimensionless).
Note that this expression is dimensional so values must be entered in the units specified.
Values for ΦS shall be established from Figure 751.38.4.2 based on the coefficient of variation of the mean uniaxial compressive strength, , determined in accordance with methods described in EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation for the site and location in question. Values for v can be estimated from Table 751.38.4.1.
For cases where the footing is underlain by practically homogeneous rock masses, H can be assumed to be equal to the footing dimension, B and values for , and v shall be taken as the mean values of these parameters for the rock mass between the base of the footing and a depth of 2∙H below the base of the footing. For cases where the rock beneath the footing is stratified, the value for H can be assumed to be the cumulative thickness of the more compressible strata within a depth of 2∙B beneath the base of the footing. In such cases, values for , and v shall be taken as the mean values of these parameters over the thickness of the more compressible strata.
751.38.4.3 Settlement of Spread Footings on Cohesive Soils
| Commentary for EPG 751.38.4.3 Settlement of Spread Footings on Cohesive Soils |
Evaluation of settlement for spread footings on cohesive soils requires an iterative approach because analytic expressions for the minimum dimensions cannot be derived as is the case for settlement of footings on rock. As such, the procedure for evaluating settlement of footings in cohesive soils requires comparison of a factored settlement computed for the greatest minimum footing dimensions established for the strength limit states according to EPG 751.38.3 with an established tolerable settlement. If the factored total settlement determined from these provisions is found to be less than or equal to the tolerable settlement, i.e. if
| (consistent units of length) | Equation 751.38.4.3 |
where:
- δR = factored total settlement (consistent units of length) and
- δtol = tolerable settlement (consistent units of length),
the limit state is satisfied and the probability of footing settlement exceeding the tolerable settlement is less than or equal to the target probability established by MoDOT. If the factored total settlement is determined to exceed the tolerable settlement, the probability of footing settlement exceeding the tolerable value is greater than the target probability established by MoDOT. In such cases, the footing dimensions shall be increased until the factored total settlement is less than or equal to the tolerable settlement.
Resistance factors provided in this article were established to produce factored settlements that have a target probability of being exceeded. Target probabilities of exceedance were established by MoDOT for structures of different operational importance. Additional information regarding development of the resistance factors and application of the resistance factors for settlement calculations are provided in the commentary that accompanies these guidelines.
Tolerable settlement
For this provision, the tolerable settlement shall be taken as
| (consistent units of length) | Equation 751.38.4.4 |
where:
- S = length of shortest bridge span adjacent to footing (consistent units of length).
Factored total settlement
The factored settlement for footings on cohesive soils shall be computed following classical consolidation theory (e.g. Reese et al., 2006), modified to include resistance factors to be applied to the compression and recompression indices, cc and cr, and to the maximum past vertical effective stress, σ'p (also referred to as the pre-consolidation stress). Application of this method within the LRFD framework requires comparison of a factored value for σ'p, with the initial and final vertical effective stresses, σ'0 and σ'f.
If σ'0 < Φp σ'p < σ'f , the factored total settlement shall be computed as:
| (consistent units of length) | Equation 751.38.4.5 |
where:
- σ'0 = initial vertical effective stress (consistent units of stress),
- Φp = resistance factor to be applied to pre-consolidation stress (dimensionless),
- σ'p = maximum past vertical effective stress or pre-consolidated stress (consistent units of stress),
- σ'f = final vertical effective stress (consistent units of stress),
- δR = factored settlement (consistent units of length),
- H0 = thickness of compressible layer (consistent units of length),
- e0 = initial void ratio (dimensionless),
- cc = compression index (dimensionless),
- Φc = resistance factor to be applied to compression index term (dimensionless),
- cr = recompression index (dimensionless), and
- Φr = resistance factor to be applied to recompression index term (dimensionless).
If Φp σ'p ≥ σ'f, the factored settlement shall be computed as:
| (consistent units of length) | Equation 751.38.4.6 |
Similarly, if Φp σ'p ≤ σ'0, the factored settlement shall be computed as:
| (consistent units of length) | Equation 751.38.4.7 |
Values for Φc and Φr shall be established from Figure 751.38.4.3.1 based on the coefficient of variation of the mean compression index () and mean recompression index (), respectively. Similarly, values for Φp shall be established from Figure 751.38.4.3.2 based on the coefficient of variation of the mean maximum past vertical effective stress (). Coefficients of variation for each of these parameters shall be determined in accordance with methods described in EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation.
Where footings are underlain by compressible soils of substantial thickness, the soil beneath the footing shall be subdivided into several sublayers to account for potential changes in consolidation parameters and stress distribution beneath the footing. Compression of each of these sublayers shall be computed using Equation 751.38.4.5, 751.38.4.6 or 751.38.4.7, as appropriate, and the resulting values should be summed to arrive at the total settlement. For each sublayer, values for cc, cr and e0 shall be taken as the mean values of these parameters over the thickness of the sublayer. Values for H0 shall be taken as the thickness of the respective sublayer. Values for σ'0, σ'f and σ'p for each sublayer shall also be taken as the mean values over each sublayer, although this is often approximated by using values calculated for the center of the sublayer. Values used for , and shall be representative of the variability and uncertainty of the mean values for the respective parameters within each sublayer.
Where conditions warrant, settlement contributions due to immediate elastic settlement and secondary compression shall be added to those computed from Equations 751.38.4.5, 751.38.4.6 or 751.38.4.7.
751.38.4.4 Settlement of Spread Footings on Cohesionless Soils
| Commentary for EPG 751.38.4.4 Settlement of Spread Footings on Cohesionless Soils |
Spread footings in cohesionless soils shall be designed according to current AASHTO LRFD Bridge Design Specifications.
751.38.5 Modifications for Load Eccentricity
The minimum footing dimensions established in accordance with EPG 751.38.3 and EPG 751.38.4 must be increased to account for load eccentricity when resultant factored column loads are not located at the center of the footing. Furthermore, the eccentricity of factored loads on spread footings shall be restricted to prevent overturning of foundations or excessively high localized stresses at the edges of the footing as provided in this subarticle.
Load eccentricity shall be calculated in the width and length dimension directions as:
| (consistent units of length) | Equation 751.38.5.1 | |
| (consistent units of length) | Equation 751.38.5.2 |
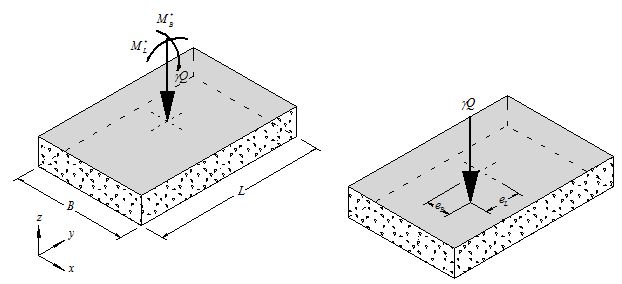
where and are moments attributed to factored load effects in the B and L directions (consistent units of force times length), respectively, and is the resultant factored load (consistent units of force) for the strength limit state (Figure 751.38.5.1). Here the moment, , is a moment about the y-axis and moment, , is a moment about the x-axis.
751.38.5.1 Modifications to Footing Dimensions for Eccentric Loads
In cases where spread footings will be subjected to eccentric loads, the minimum footing dimensions established in accordance with EPG 751.38.3 and EPG 751.38.4 shall be determined using reduced dimensions, B' and L' , instead of the actual dimensions, B and L, where
| B' = B - 2eB | (consistent units of length) | Equation 751.38.5.3 |
| L' = L - 2eL | (consistent units of length) | Equation 751.38.5.4 |
where eB and eL are the load eccentricity due to the factored load in the width and length dimensions, respectively.
751.38.5.2 Limiting Eccentricity in Soil and Cohesive Intermediate Geomaterials
For footings founded in soil or cohesive intermediate geomaterials, the load eccentricity shall be restricted to the middle one-half of the footing. Minimum footing dimensions satisfying this criterion are:
| B ≥ 4eB and L ≥ 4eL | (consistent units of length) | Equation 751.38.5.5 |
751.38.5.3 Limiting Eccentricity in Cohesionless Intermediate Geomaterials and Rock
For footings founded in cohesionless intermediate geomaterials and rock, the load eccentricity shall be restricted to the middle three-quarters of the footing. Minimum footing dimensions satisfying this criterion are:
| and | (consistent units of length) | Equation 751.38.5.6 |
751.38.6 Design for Lateral Loading
| Commentary for EPG 751.38.6 Design for Lateral Loading |
Spread footings subjected to substantial lateral loads shall be designed according to the lateral load provisions of current AASHTO LRFD Bridge Design Specifications, including consideration of sliding stability.
751.38.7 Design for Overall Stability
Overall stability shall be evaluated when spread footings are located near to an embankment, excavated, or natural slope. Overall stability shall be evaluated at the Service I limit state. Overall stability shall be evaluated using methods described in EPG 321.1 Design of Earth Slopes for evaluation of slope stability with the factored footing loads applied as a surcharge load.
751.38.8 Structural Design of Spread Footings
| Commentary for EPG 751.38.8 Structural Design of Spread Footings |
The provisions provided in this subarticle are unchanged from prior versions of the EPG aside from minor editorial revisions.
Structural design and detailing of spread footings should be accomplished considering the shear and moment capacity of the footing when subjected to factored column loads.
751.38.8.1 Design for Shear
The footing shall be designed so that the shear strength of the concrete is adequate to handle the shear stress without the additional help of reinforcement. If the shear stress is too great, the footing depth should be increased.
The shear capacity of the footings in the vicinity of concentrated loads shall be governed by the more severe of the following two conditions.
751.38.8.1.1 One-Way Shear
Critical sections shall be taken from the face of the column for square or rectangular columns or at the equivalent square face of a round column. The equivalent square column is the column which has a cross-sectional area equal to the round section of the actual column and placed concentrically as shown in Figure 751.38.8.1.
One-Way Shear Capacity shall be evaluated as:
| (consistent units) | Equation 751.38.8.1 |
where:
- Φ = 0.9
- Vn = Vc =
- B = footing width
- β = factor indicating ability of diagonally cracked concrete to transmit tension = 2.0
- dv = effective shear depth of concrete
- Vu =
- vu = the triangular or trapezoidal stress distribution applied to the designated loaded area of the footing from the strength limit state load combination
751.38.8.1.2 Two-Way Shear
The critical section for checking Two-Way Shear is taken from the boundary of a square area with sides equal to the equivalent square column width plus the effective shear depth as shown.
Two-Way Shear Capacity shall be evaluated as:
| = | (consistent units) | Equation 751.38.8.1 |
where:
- ΦVn =
- βc = ratio of long side to short side of the rectangle through which the concentrated load or reaction force is transmitted,
- bo = perimeter of critical section = 4(dv + equivalent square column width),
- dv = effective shear depth of concrete (inches)
- Vu = maximum axial load on top of footing from column reactions for strength limit state load combinations
Table 751.38.8.1 shows approximate capacities for both One-Way and Two-Way Shear for the given footing depth and column diameter to assist in selecting a footing length and width.
Table 751.38.8.1 Shear Capacities for Given Column Diameters and Footing Depths
| Column Diameter (ft) |
Footing Depth (ft) |
One-Way Shear Capacity, Vr kip/ft |
Two-Way Shear Capacity, Vr kips |
|---|---|---|---|
| 2.5 | 2.50 | 30.7 | 1074 |
| 2.5 | 2.75 | 34.3 | 1266 |
| 2.5 | 3.00 | 37.8 | 1473 |
| 2.5 | 3.25 | 41.4 | 1694 |
| 2.5 | 3.50 | 44.9 | 1928 |
| 2.75 | 2.75 | 34.3 | 1327 |
| 2.75 | 3.00 | 37.8 | 1540 |
| 2.75 | 3.25 | 41.4 | 1767 |
| 2.75 | 3.50 | 44.9 | 2008 |
| 2.75 | 3.75 | 48.5 | 2263 |
| 3.00 | 3.00 | 37.8 | 1607 |
| 3.00 | 3.25 | 41.4 | 1840 |
| 3.00 | 3.50 | 44.9 | 2087 |
| 3.00 | 3.75 | 48.5 | 2348 |
| 3.00 | 4.00 | 52.0 | 2624 |
| 3.25 | 3.25 | 41.4 | 1913 |
| 3.25 | 3.50 | 44.9 | 2166 |
| 3.25 | 3.75 | 48.5 | 2434 |
| 3.25 | 4.00 | 52.0 | 2716 |
| 3.25 | 4.25 | 55.6 | 3012 |
| 3.50 | 3.50 | 44.9 | 2246 |
| 3.50 | 3.75 | 48.5 | 2520 |
| 3.50 | 4.00 | 52.0 | 2808 |
| 3.50 | 4.25 | 55.6 | 3110 |
| 3.50 | 4.50 | 59.1 | 3426 |
| 3.75 | 3.75 | 48.5 | 2605 |
| 3.75 | 4.00 | 52.0 | 2900 |
| 3.75 | 4.25 | 55.6 | 3208 |
| 3.75 | 4.50 | 59.1 | 3531 |
| 3.75 | 4.75 | 62.7 | 3868 |
| 4.00 | 4.00 | 52.0 | 2992 |
| 4.00 | 4.25 | 55.6 | 3306 |
| 4.00 | 4.50 | 59.1 | 3635 |
| 4.00 | 4.75 | 62.7 | 3978 |
| 4.00 | 5.00 | 66.2 | 4335 |
| 4.25 | 4.25 | 55.6 | 3404 |
| 4.25 | 4.50 | 59.1 | 3740 |
| 4.25 | 4.75 | 62.7 | 4089 |
| 4.25 | 5.00 | 66.2 | 4452 |
| 4.25 | 5.25 | 69.8 | 4830 |
| 4.50 | 4.50 | 59.1 | 3844 |
| 4.50 | 4.75 | 62.7 | 4200 |
| 4.50 | 5.00 | 66.2 | 4569 |
| 4.50 | 5.25 | 69.8 | 4953 |
| 4.50 | 5.50 | 73.3 | 5351 |
| 4.75 | 4.75 | 62.7 | 4310 |
| 4.75 | 5.00 | 66.2 | 4686 |
| 4.75 | 5.25 | 69.8 | 5076 |
| 4.75 | 5.50 | 73.3 | 5481 |
| 4.75 | 5.75 | 76.8 | 5899 |
| 5.00 | 5.00 | 66.2 | 4803 |
| 5.00 | 5.25 | 69.8 | 5200 |
| 5.00 | 5.50 | 73.3 | 5610 |
| 5.00 | 5.75 | 76.8 | 6035 |
| 5.00 | 6.00 | 80.4 | 6474 |
| 5.25 | 5.25 | 69.8 | 5323 |
| 5.25 | 5.50 | 73.3 | 5740 |
| 5.25 | 5.75 | 76.8 | 6171 |
| 5.25 | 6.00 | 80.4 | 6616 |
| 5.50 | 5.50 | 73.3 | 5869 |
| 5.50 | 5.75 | 76.8 | 6306 |
| 5.50 | 6.00 | 80.4 | 6758 |
| 5.75 | 5.75 | 76.8 | 6442 |
| 5.75 | 6.00 | 80.4 | 6900 |
| 6.00 | 6.00 | 80.4 | 7042 |
- Assumptions:
- Φ = 0.9
- = 3 ksi
- β = 2.0
- dv = footing depth: 4 inches
- One-Way Shear Capacity =
- Where One-Way Shear capacity is per foot width of footing, i.e. where total shear capacity is Total Vr = Vr from table × B
- Two-Way Shear Capacity =
751.38.8.2 Moment
The critical section for bending shall be taken at the face of the equivalent square column. The applied moment shall be determined from a triangular or trapezoidal stress distribution on the bottom of the footing.
The bearing pressure used to design bending reinforcement shall be calculated from Strength I, III, IV and V Load Combinations.
Reinforcement must meet the maximum and minimum requirements as given in LRFD 5.7.3.3.1 and LRFD 5.7.3.3.2.
The minimum reinforcement allowed is #5 bars spaced at 12”.
751.38.8.2.1 Distribution of Reinforcement
Reinforcement in the long direction shall be distributed uniformly across the entire width of footing.
For reinforcement in the short direction, a portion of the total reinforcement shall be distributed uniformly over a band width equal to the length of the short side of footing and centered on the centerline of column or pier as shown in Figure 751.38.8.3.
The band width reinforcement required shall be calculated by the following equation:
| Equation 751.38.8.3 |
where:
- A(s-BW) = area of steel in the band width (in2),
- A(s-SD) = total area of steel in short direction (in2),
- β = ratio of the long side to the short side of footing
The remainder of the reinforcement required in the short direction shall be distributed uniformly outside the center band width of footing.
751.38.8.2.2 Crack Control Reinforcement
The reinforcement shall meet the spacing criteria, s, as specified.
where:
- βs = ,
- dc = concrete cover measured from extreme tension fiber to center of flexural reinforcement (in.),
- fs = tensile stress in reinforcement at the service limit state (ksi),
- h = depth of footing (in.)
- = 1.0 for Class 1 exposure condition
751.38.8.3 Details
751.38.8.3.1 Spread Footing Reinforcement
| File:751.38 Reinforcement Front Elevation.gif | File:751.38 Reinforcement Side Elevation.gif |
| FRONT ELEVATION | SIDE ELEVATION |
| Fig. 751.38.8.4 Schematic showing typical reinforcement detail in front elevation and side elevation. | |
| * Footing depths > 36 in. may require the side faces to have shrinkage and temperature reinforcement. See Structural Project Manager. | |
751.38.9 References
AASHTO (2009), AASHTO LRFD Bridge Design Specification: Customary U.S. Units, American Association of State Highway and Transportation Officials, Fourth Edition with 2008 and 2009 Interim Revisions.
Hoek, E., and E.T. Brown (1988), “The Hoek-Brown Failure Criterion – A 1988 Update,” Proceedings of the 15th Canadian Rock Mechanics Symposium, Toronto, Canada.
Kulhawy, F.H. (1978), “Geomechanical Model for Rock Foundation Settlement,” Journal of the Geotechnical Engineering Division, ASCE, Vol. 104, No. GT2, pp. 211-227.
Marinos, P., and E. Hoek (2000), “GSI: A Geologically Friendly Tool for Rock Mass Strength Estimation,” Proceedings of GeoEng2000, Melbourne, Australia, Vol. I, pp. 1422-1440.
Reese, L.C., W.M. Isenhower, and S-T Wang (2006), Analysis and Design of Shallow and Deep Foundations, John Wiley and Sons, 574 pp.
Wyllie, D.C. (1999), Foundations on Rock, E & FN Spon, Second Edition, 401 pp.
751.38.10 Commentary
Commentary on EPG 751.38.1 General
These guidelines were developed from prior EPG guidelines with notable changes to the general approach for application of LRFD techniques as well as updated resistance factors based on probabilistic calibrations. Calibration analyses were performed following generally accepted procedures for calibration of resistance factors for geotechnical applications, but with modifications to permit several enhancements to be included in the guidelines. The most notable enhancements provided in the guidelines include:
- Use of resistance factors that are contingent upon the variability and uncertainty that exists in select design properties.
- Adoption of different target reliability levels for foundations of structures of different operational importance.
Both of these enhancements are expected to produce efficient foundation designs while still maintaining appropriate safety and reliability for all classes of operational importance. Additional information regarding development of the methods provided in these guidelines can be found in Abu El-Ela et al. (2011) and Song et al. (2011). Additional information regarding target reliability values established for different classes of operational importance is provided in Bowders et al. (2011).
The four classes of operational importance include:
- Minor and low volume route
- Major route
- Major bridge costing less than $100 million
- Major bridge costing greater than $100 million.
These classifications are based on common MoDOT designations. The target reliability levels established for each limit state and operational importance were generally based upon consideration of highway bridges. However, the methods in this article can also be utilized for design of foundations for other structures including retaining walls and roadway signs.
Calibration analyses performed to establish the resistance factors presented in this subarticle were performed using the latest knowledge of variability and uncertainty of applied loads (Kulicki et al., 2007), as well as using load factors that are currently in effect. The resistance factors provided in these guidelines are intended to produce foundations with reliabilities that are approximately equal to the target reliabilities established by MoDOT when utilized with current load factors. Since it is the combined effect of load and resistance factors that produce this reliability, the resistance factors provided are inherently coupled with current load factors and are contingent upon the uncertainty and variability in the applied loads that was presumed for the calibrations. As such, recalibration of resistance factors is required if alternative load factors are adopted, or if substantial revisions to current estimates of load variability and uncertainty are found.
It is important to emphasize that the resistance factors provided in these guidelines were developed presuming that mean values would be used for all design parameters in the methods provided. This departs from past practice utilizing allowable stress design (ASD) approaches where nominal values of parameters that were less than mean values were often used to introduce conservatism into the analyses beyond that provided by the ASD factor of safety. Use of design parameters less than the mean values within the context of these guidelines will often, but not always, increase the reliability of foundation designs; however, such practice is contrary to the spirit of LRFD in that it will not produce foundations that achieve the target reliability established by MoDOT policy.
The procedures provided in these guidelines are not intended as a substitute for good judgment. Rather, the intent of these guidelines is to:
- 1) inform designers of generally appropriate levels of conservatism to address variability and uncertainty involved in different aspects of design analyses and
- 2) provide quantitative methods to achieve target reliabilities for foundations depending on the variability and uncertainty present in relevant design parameters and design methods.
Designers must still use their best judgment in considering design options (e.g. foundation depth, type, and size; necessity for load tests; etc.) for establishing the most appropriate foundations for bridges and other structures.
Commentary on EPG 751.38.1.2 General Design Considerations
When considering placement of spread footings within the prohibited region of Figure 751.38.1.2, evaluations of overall stability shall be performed in accordance with EPG 751.38.7.
The prohibited region for rock slopes varies with the quality of the rock present at a site and other factors. As a general rule of thumb, the limit line is inclined at 1:1 (V:H). However, the line may be flatter for particularly poor rock and steeper for particularly good rock.
Commentary on EPG 751.38.2 General Design Procedure and Limit States
Selection of applicable strength and serviceability limit states shall be accomplished in close consultation with the Structural Project Manager. At a minimum, the Strength I and Service I limit states should be evaluated. When multiple strength and/or service limit states are considered, the limit state producing the greatest minimum footing dimensions shall govern the final design dimensions.
Commentary on EPG 751.38.3 Design for Axial Loading at Strength Limit States
Throughout EPG 751.38, factored loads are denoted as . This notation should not be taken to suggest inclusion or exclusion of specific load effects, but rather is simply intended as a convenient notation to reflect factored loads. When applying these guidelines, designers should replace with load combinations and load factors that are appropriate for the structure and limit state being considered.
Design procedures within this subarticle are categorized according to material type, including methods for design of spread footings founded upon “rock”, “weak rock”, “cohesive soil” and “cohesionless soil”. While these categories serve to logically separate the guidelines according to design method, complexities present at some sites may lead to cases where multiple methods could potentially be used. In such cases, designers should utilize the method that is most appropriate for the conditions encountered, rather than selecting the method that produces the smallest or largest footing dimensions.
EPG 751.38.3.1 is generally intended for use with “harder” rock materials where the frequency, orientation, and condition of rock discontinuities tend to dominate the response of the rock to loading from foundations. Such rock masses will generally be composed of rock with uniaxial compressive strengths that are greater than 100 ksf, although some exceptions to this limit could arise. Limestones and dolomites will commonly fall under this subarticle as will many sandstones, and even a few hard shales.
EPG 751.38.3.2 is intended for use with weaker rock where the properties of the intact rock tend to dominate performance. This subarticle is primarily intended for use with shales, some weak sandstones, and potentially some very stiff clays. Use of methods provided in EPG 751.38.3.2 for materials with uniaxial compressive strengths greater than 100 ksf should be done with extreme caution as the methods may dramatically overestimate the bearing resistance that can be realistically achieved for rock with greater uniaxial compressive strengths.
EPG 751.38.3.3 and EPG 751.38.3.4 are intended to use with cohesive and cohesionless soils, respectively. The methods provided in EPG 751.38.3.3 are in fact similar to those provided for weak rock in EPG 751.38.3.2, except that the uniaxial compressive strength used in EPG 751.38.3.2 is replaced by the undrained shear strength in EPG 751.38.3.3 according to conventions of practice. Some overlap exists between the strength limits provided in EPG 751.38.3.2 and EPG 751.38.3.3. (Note that the limits for EPG 751.38.3.2 are based on the uniaxial compressive strength whereas the limits for EPG 751.38.3.3 are based on the undrained shear strength, which is nominally one half of the compressive strength.) When designing for materials that fall within this overlapping range of strengths, designers shall use the method that is most appropriate for the material encountered.
Commentary on EPG 751.38.3.1 Bearing Resistance for Spread Footings on Rock (qu ≥ 100 ksf)
The design method provided in this subarticle is adapted from the method presented in Wyllie (1999) to conform to the LRFD approach. The method is derived from the Hoek-Brown strength criterion (Hoek and Brown, 1988) that is commonly used to represent the strength of fractured rock masses using the rock mass parameters, m and s. The resistance factors provided in Figure 751.38.3.1 were established from probabilistic calibrations to achieve the target foundation reliabilities as described in Abu El-Ela et al. (2011). These calibrations were conducted with explicit consideration of variability and uncertainty present for dead load, live load, uniaxial compressive strength, and the design method itself (i.e. a “method” uncertainty). The variability and uncertainty utilized for dead load and live load were taken from Kulicki et al. (2007). The variability and uncertainty in the design method were conservatively estimated utilizing the likely range of m and s values expected for a particular condition.
Unfortunately, empirical data to evaluate design methods for predicting the bearing resistance of footings on fractured rock are not presently available. As such, the variability and uncertainty attributed to the design method was conservatively estimated as a matter of prudence. One consequence of this conservatism is that the factored resistance predicted for foundations designed according to EPG 751.38.3.1 may, in some cases, be less than the factored resistance predicted according to EPG 751.38.3.2 for rock that might be considered to have lower quality. This consequence is a reflection of the lack of data available to confirm the predicted resistance using the prescribed method, and thus the limited reliability of the method, rather than an indication that the bearing resistance will actually be less than that for lesser rock. Future research to measure the ultimate bearing resistance of foundations in fractured rock could dramatically improve the accuracy and reliability of these methods, which in turn would dramatically improve the efficiency of foundations in fractured rock. This consequence also suggests that site specific load tests could potentially improve foundation efficiency in some cases while still maintaining the target reliability.
The coefficient of variation for the mean uniaxial compressive strength used in Equation 751.38.3.3 shall reflect the variability and uncertainty in the mean compressive strength rather than the variability and uncertainty in measurements of compressive strength as described in EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation and the associated commentary. Values for qu and , m, and s do not have to be established exclusively based on tests or observations located within the depth range of interest below the footing. However, the values used should reflect the mean and variability in the material parameters within that depth range.
Several methods are available for establishing appropriate values of GSI for specific rock masses. Equation 751.38.3.6 represents a generally rigorous approach for determination of GSI that should be used when available measurements and observations allow for establishing Rock Mass Rating system ratings and when these ratings produce RMR greater than 25. In cases where such measurements and observations are not available, or where RMR is less than 25, GSI values can be estimated using the qualitative chart shown in Fig. Commentary 751.38.3.1.1 based on the work of Marinos and Hoek (2000). Figs. Commentary 751.38.3.1.2, Commentary 751.38.3.1.3, and Commentary 751.38.3.1.4 provide additional guidance for qualitative selection of GSI for typical sandstones, shales, and limestones from the chart.
In cases where GSI cannot be rationally determined, it is also possible to directly estimate approximate values for the rock mass parameters m and s from Table Commentary 751.38.3.1 using qualitative descriptions of the rock mass. The values provided in Table Commentary 751.38.3.1 will generally be less than values that will be produced using Equations 751.38.3.4 and 751.38.3.5. This result is because the values in Table Commentary 751.38.3.1 were established under the assumption that excavation-induced damage will occur (i.e. that the Hoek and Brown damage factor, D, is equal to 1) while Equations 751.38.3.4 and 751.38.3.5 were established assuming that no significant excavation-induced damage will occur (i.e. that D = 0). Since significant excavation-induced damage is unlikely to occur for footings excavated using conventional construction techniques, the values provided in Table Commentary 751.38.3.1 will be conservative. It is also important to point out that m and s can be roughly interpolated from the values provided in Table Commentary 751.38.3.1 for conditions falling between those listed.
Methods provided in this subarticle are not appropriate for use with uniaxial compressive strengths estimated from Point Load Index tests or from other empirical correlations. Use of correlations for estimation of uniaxial compressive strength introduces additional variability into the relation among rock mass parameters, uniaxial compressive strength, and bearing resistance that is not accounted for in the resistance factors provided. Use of compressive strengths derived from Point Load Index values or other correlations is therefore not appropriate for application of the provisions of this subarticle. It is possible to develop resistance factors that would be appropriate for such use, but such calibrations have not been completed at this time.
Some iteration may be required for the Cf1 term in Equation 751.38.3.3. Application of Equation 751.38.3.3 requires an assumption regarding the shape of the spread footing to establish the required footing dimensions. If that assumption must be changed, either as a result of design calculations or other considerations, Equation 751.38.3.3 shall be re-evaluated to ensure that the provision remains satisfied.
Commentary on EPG 751.38.3.2 Bearing Resistance for Spread Footings on Weak Rock (5 ksf ≤ qu ≤ 100 ksf)
The design method provided in this article is adapted from methods presented in Wyllie (1999) to conform to the LRFD approach. The method is derived from the classical bearing capacity equation. The resistance factors provided in Figure 751.38.3.2 were established from probabilistic calibrations to achieve the target foundation reliabilities as described in Abu El-Ela et al. (2011). These calibrations were conducted with explicit consideration of variability and uncertainty present for dead load, live load, and uniaxial compressive strength in addition to the variability and uncertainty present in the method itself. The variability and uncertainty utilized for dead load and live load were taken from Kulicki et al. (2007). Variability and uncertainty for the method was conservatively estimated based on consideration of the range of potential values for the actual bearing capacity factor including the effects of the correction factors provided in Equations 751.38.3.8, 751.38.3.9 and 751.38.3.10.
The coefficient of variation for the mean uniaxial compressive strength used in Equation 751.38.3.7 shall reflect the variability and uncertainty in the mean compressive strength rather than the variability and uncertainty in measurements of compressive strength as described in EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation. Values for and do not have to be established exclusively based on tests or observations located within the depth range of interest below the footing. However, the values used should reflect the mean and variability in the material parameters within that depth range.
Methods provided in this subarticle are not appropriate for use with uniaxial compressive strengths estimated from Point Load Index tests or from other empirical correlations. Use of correlations for estimation of uniaxial compressive strength introduces additional variability into the relation among rock mass parameters, uniaxial compressive strength, and bearing resistance that is not accounted for in the resistance factors provided. Use of compressive strengths derived from Point Load Index values or other correlations is therefore not appropriate for application of the provisions of this subarticle. It is possible to develop resistance factors that would be appropriate for such use, but such calibrations have not been completed at this time.
Commentary on EPG 751.38.3.3 Bearing Resistance for Spread Footings on Cohesive Soils (su ≤ 5,000 psf)
Resistance factors provided in Figure 751.38.3.3 for bearing resistance of spread footings on cohesive soils are identical to those provided in Figure 751.38.3.2. The only differences in the methods presented in EPG 751.38.3.2 and EPG 751.38.3.3 is that EPG 751.38.3.2 is presented in terms of the uniaxial compressive strength while EPG 751.38.3.3 is presented in terms of the undrained shear strength.
The coefficient of variation for the mean undrained shear strength used in Equation 751.38.3.11 shall reflect the variability and uncertainty in the mean shear strength rather than the variability and uncertainty in measurements of shear strength as described in EPG 321.3 Procedures for Estimation of Geotechnical Parameter Values and Coefficients of Variation. Values for and do not have to be established exclusively based on tests or observations located within the depth range of interest below the footing. However, the values used should reflect the mean and variability in the material parameters within that depth range.
The resistance factors provided in this subarticle are based on the assumption that measurements of undrained shear strength will accurately reflect the actual undrained shear strength in the field. Use of undrained shear strength values established from approximations or from index tests such as hand-held penetrometer tests, Torvane tests, or Standard Penetration Tests will introduce additional variability and uncertainty into the design that is currently not reflected in the resistance factors provided. As such, it is not generally appropriate to use such approximations for estimating undrained shear strength for use in these provisions. At a minimum, undrained shear strengths should be established based on unconfined compression tests performed on specimens acquired using good quality boring techniques and good quality “undisturbed” sampling with thin walled samplers. It is preferable to perform unconsolidated-undrained type triaxial tests or consolidated-undrained type triaxial tests to establish undrained shear strength values for use in these provisions.
Commentary on EPG 751.38.3.4 Bearing Resistance for Spread Footings on Cohesionless Soils
Probabilistic calibrations for spread footings on cohesionless soils have not yet been completed by MoDOT. The provisions of current AASHTO LRFD Bridge Design Specifications should therefore be followed when designing spread footings on cohesionless soils.
Commentary on EPG 751.38.4 Design for Axial Loading at Serviceability Limit States
The provisions of this subarticle were developed to limit foundation settlements to be less than generally tolerable levels of settlement with some target reliability. Target reliability levels for service limit states are substantially less than target reliability levels for strength limit states because the consequences associated with serviceability limit states are substantially less than consequences for strength limit state conditions. The ramification of these facts is that some foundations designed according to these provisions may experience settlements that exceed tolerable settlements in some instances. The frequency of foundations settling more than tolerable limits should approach the established target probabilities of exceedance when considered over a large number of projects. In cases where actual foundation settlements are observed to exceed tolerable limits, appropriate remedial measures shall be applied to the foundation(s) and/or the structure that it is supporting so that appropriate reliability is maintained.
Tolerable settlements used throughout these provisions were established from theoretical considerations and empirical observations of bridge performance based on the work of Moulton (1984) and Duncan and Tan (1991). Three different serviceability conditions corresponding to different levels of required maintenance and repair were initially considered:
- 1) minor damage generally corresponding to the theoretical onset of deck cracking (Duncan and Tan, 1991),
- 2) more significant damage corresponding to the onset of structural distress based on empirical observations by Moulton (1986) and
- 3) major damage corresponding to theoretical overstress of the bridge superstructure (Moulton, 1986).
Target reliabilities for each of these conditions were established based on economic analyses described in Bowders et al. (2011). Comparative analyses for typical design conditions were then performed to evaluate the alternative serviceability conditions. Results of these analyses generally indicate that the first serviceability condition, corresponding to minor damage, tends to control footing dimensions. These guidelines therefore only require evaluation of this condition (the others being presumed to be inherently satisfied based on the analyses performed).
Based on this work, tolerable settlements are established according to an angular distortion, defined as
| (consistent units of stress) | Equation Commentary 751.38.4.1 |
where:
- A = angular distortion (dimensionless),
- ∆ = differential settlement between adjacent footings (consistent units of length),
- S = span between adjacent footings (consistent units of length).
This limiting value of angular distortion is based on theoretical consideration of the onset of deck cracking (Duncan and Tan, 1991). This limit is implicitly included in the methods provided in EPG 751.38.4.1 and EPG 751.38.4.2, while it is explicitly included in EPG 751.38.4.3.
The target probabilities of exceedance reflected in the resistance factors provided in EPG 751.38 correspond to the target values established by MoDOT based on economic considerations. While use of alternative limits for tolerable settlement is possible, such use is not strictly appropriate since the target probabilities adopted by MoDOT for different classes of operational importance were established based on consequences associated with the limit provided in Equation Commentary 751.38.4.1. Other limits would generally require different target probabilities, and thus different resistance factors to achieve the same economic balance.
As was the case in EPG 751.38.3, design procedures within this subarticle are categorized according to material type, including methods for design of spread footings founded upon “rock”, “weak rock”, “cohesive soil”, and “cohesionless soil”. While these categories serve to logically separate the guidelines according to design method, complexities present at some sites may lead to cases where multiple methods could potentially be used. In such cases, designers should utilize the method that is most appropriate for the conditions encountered, rather than selecting the method that produces the smallest or largest footing dimensions.
EPG 751.38.4.1 is generally intended for use with “harder” rock materials where the frequency, orientation, and condition of rock discontinuities tend to dominate the response of the rock to loading from foundations. Such rock masses will generally be composed of rock with uniaxial compressive strengths that are greater than 100 ksf, although some exceptions to this limit could arise. Limestones and dolomites will commonly fall under this subarticle as will many sandstones, and even a few hard shales.
EPG 751.38.4.2 is intended for use with weaker rock where the properties of the intact rock tend to dominate performance. This subarticle is primarily intended for use with shales, some weak sandstones, and potentially some very stiff clays.
EPG 751.38.4.3 and EPG 751.38.4.4 are intended for use with cohesive and cohesionless soils, respectively.
Throughout EPG 751.38, factored loads are denoted as . This notation should not be taken to suggest inclusion or exclusion of specific load effects, but rather is simply intended as a convenient notation to reflect factored loads. When applying these guidelines, designers should replace with load combinations and load factors that are appropriate for the structure and limit state being considered.
Commentary on EPG 751.38.4.1 Settlement of Spread Footings on Rock (qu ≥ 100 ksf)
The provisions of this subarticle are derived from the conventional elastic settlement formula, incorporating estimates of rock mass modulus from Hoek and Brown (1997). The resistance factors provided in Figure 751.38.4.1 were established from probabilistic calibrations to achieve the target foundation reliabilities as described in Abu El-Ela et al. (2011). These calibrations were conducted with explicit consideration of variability and uncertainty present for dead load, live load, uniaxial compressive strength, and a “method variability” to account for variability and uncertainty introduced by the elastic model in general, and the estimates of rock mass modulus, Em, in particular. The variability and uncertainty utilized for dead load and live load were taken from Kulicki et al. (2007). The “method variability” was conservatively assumed for development of resistance factors for this provision of the guidelines because of the lack of data available upon which to judge the accuracy of the method. It is likely that this provision could be made more efficient (i.e. made to produce smaller footings) with additional study should this provision control the size of spread footings on a routine basis.
Guidance for establishing appropriate values for GSI is provided in EPG 751.38.3.1 and the associated commentary.
When the term H in Equation 751.38.4.1 is taken to be a multiple of the foundation width, B, it is possible to cancel terms on both sides of the equation to arrive at an expression for the minimum foundation length, L. Strictly speaking, the equations produce the result of a minimum L for some assumed B, but this can be done for ANY value of B. In such cases, designers should avoid “getting wrapped up in the math” to arrive at unreasonable values for B and L and remember that spread footings shall be made as close to square as possible according to the provisions of EPG 751.38.1.2.
For the purposes of this provision, use of “more compressible” strata reflects the need for the designer to judge the relative stiffness of different strata beneath the footing. If the rock beneath the footing is composed of alternating strata of relatively stiff and soft rock, the thickness H shall be taken to reflect the cumulative thickness of relatively soft rock within a depth range from the base of the footing to a depth of 2∙B below the base of the footing.
Commentary on EPG 751.38.4.2 Settlement of Spread Footings on Weak Rock (5 ksf ≤ qu ≤ 100 ksf)
The provisions of this subarticle are derived from the conventional elastic settlement formula, incorporating estimates of rock mass modulus from Rowe and Armitage (1984). The resistance factors provided in Figure 751.38.4.2 were established from probabilistic calibrations to achieve the target foundation reliabilities as described in Abu El-Ela et al. (2011). These calibrations were conducted with explicit consideration of variability and uncertainty present for dead load, live load, uniaxial compressive strength, and a “method variability” to account for variability and uncertainty introduced by the elastic model in general, and the estimates of rock mass modulus, Em, in particular. The variability and uncertainty utilized for dead load and live load were taken from Kulicki et al. (2007). The “method variability” was derived from data provided by Rowe and Armitage to reflect the variability of the relationship between uniaxial compressive strength of the intact rock and the rock mass modulus. Because the variability of the method can be assessed through empirical data, the resistance factors provided in EPG 751.38.4.2 are substantially greater than those provided in EPG 751.38.4.1 where empirical data is not available.
When the term H in Equation 751.38.4.2 is taken to be a multiple of the foundation width, B, it is possible to cancel terms on both sides of the equation to arrive at an expression for the minimum foundation length, L. Strictly speaking, the equations produce the result of a minimum L for some assumed B, but this can be done for ANY value of B. In such cases, designers should avoid “getting wrapped up in the math” to arrive at unreasonable values for B and L and remember that spread footings shall be made as close to square as possible according to the provisions of EPG 751.38.1.2.
For the purposes of this provision, use of “more compressible” strata reflects the need for the designer to judge the relative stiffness of different strata beneath the footing. If the rock beneath the footing is composed of alternating strata of relatively stiff and soft rock, the thickness H shall be taken to reflect the cumulative thickness of relatively soft rock within a depth range from the base of the footing to a depth of 2∙B below the base of the footing.
Commentary on EPG 751.38.4.3 Settlement of Spread Footings on Cohesive Soils
The provisions of this subarticle are derived from conventional one-dimensional consolidation settlement equations, adapted to conform to the LRFD approach. The resistance factors provided in Figs. 751.38.4.3.1 and 751.38.4.3.2 were established from probabilistic calibrations to achieve the target foundation reliabilities as described in Song et al. (2011). These calibrations were conducted with explicit consideration of variability and uncertainty present for dead load, live load, soil compression index (cc), soil recompression index (cr), initial void ratio (eo), maximum past vertical effective stress (σ'p), and the change in effective stress due to the applied load from the foundation. A “method variability” was also included in the calibrations to reflect general variability and uncertainty associated with predictions of settlement in cohesive soils. The variability and uncertainty utilized for dead load and live load were taken from Kulicki et al. (2007). The variability in the initial void ratio was taken from analyses of site characterization data from several different sites (Likos et al., 2011). The “method variability” was established from judgment regarding the expected accuracy of the general settlement equation.
Separate resistance factors were applied to the compression and recompression indices and the maximum past vertical effective stress so that the variability of these parameters could be addressed separately. It is possible to develop a single resistance factor to be applied to the entire expression. However, such an implementation prevents individual accounting for variability in these parameters and ultimately leads to conservatism that is not necessary when the resistance factors are separated.
For spread footings on cohesive soils, elastic settlement is generally small relative to settlement arising from consolidation or secondary compression. Secondary compression can be significant, particularly in highly organic soils, but is generally small relative to consolidation settlements for purely mineral soils.
Commentary on EPG 751.38.4.4 Settlement of Spread Footings on Cohesionless Soils
Probabilistic calibrations for spread footings on cohesionless soils have not yet been completed by MoDOT. The provisions of current AASHTO LRFD Bridge Design Specifications should therefore be followed when designing spread footings on cohesionless soils.
Commentary on EPG 751.38.6 Design for Lateral Loading
Probabilistic calibrations for spread footings subjected to lateral loads have not yet been completed by MoDOT. The provisions of current AASHTO LRFD Bridge Design Specifications should therefore be followed when designing spread footings on cohesionless soils.
Commentary on EPG 751.38.8 Structural Design of Spread Footings
The provisions of this subarticle are unchanged from previous version except for minor editorial revisions.
Commentary on EPG 751.38.9 References
AASHTO (2009), AASHTO LRFD Bridge Design Specification: Customary U.S. Units, American Association of State Highway and Transportation Officials, Fourth Edition with 2008 and 2009 Interim Revisions.
Abu El-Ela, A.A., J.J. Bowders, and J.E. Loehr (2011), Calibration of LRFD Resistance Factors for Design of Spread Footings in Hard and Soft Rock, Missouri Department of Transportation, OR11.XXX, XXX pp. (in preparation)
Bowders, J.J., J.E. Loehr, and D. Huaco (2011), MoDOT Transportation Geotechnics Research Program: Development of Target Reliabilities for MoDOT Bridge Foundations and Earth Slopes, Missouri Department of Transportation, OR11.XXX, XX pp. (in preparation)
Duncan, J.M., and C.K. Tan (1991), Part 5 – Engineering Manual for Estimating Tolerable Movements of Bridges, in Manuals for the Design of Bridge Foundations, by R.M. Barker, J.M. Duncan, K.B. Rojiani, P.S.K. Ooi, C.K. Tan, and S.G. Kim, NCHRP Report 343, TRB, pp. 219-228.
Hoek, E., and E.T. Brown (1988), “The Hoek-Brown Failure Criterion – A 1988 Update,” Proceedings of the 15th Canadian Rock Mechanics Symposium, Toronto, Canada.
Hoek, E., and E.T. Brown (1997), “Practical Estimates of Rock Mass Strength,” International Journal of Rock Mechanics and Mining Sciences, Vol. 34, No. 8, 1997, pp. 1165-1186.
Kulicki, J.M., Z. Prucz, C.M. Clancy, D.R. Mertz, and A.S. Nowak (2007), Updating the Calibration Report for AASHTO LRFD Code, Final Report for NCHRP Project 20-7/186, AASHTO, 125 pp.
Likos, W.J., J.E. Loehr, N. Maerz, K.A. Magner, L. Ge, and R.W. Stephenson (2011), MoDOT Transportation Geotechnics Research Program: Site Characterization Program Interpretation Report, Missouri Department of Transportation, OR11.XXX, XXX pp. (in preparation)
Marinos, P., and E. Hoek (2000), “GSI: A Geologically Friendly Tool for Rock Mass Strength Estimation,” Proceedings of GeoEng2000, Melbourne, Australia, Vol. I, pp. 1422-1440.
Moulton, L.K. (1986), Tolerable Movement Criteria for Highway Bridges, Federal Highway Administration Report No. FHWA-TS-85-228, 93 pp.
Rowe, R.K., and H.H. Armitage (1984), The Design of Piles Socketed into Weak Rock, Geotechnical Research Report GEOT-11-84 for the National Research Council of Canada, University of Western Ontario, 366 pp.
Song, C., J.J. Bowders, A.A. Abu El-Ela, and J.E. Loehr (2011), Calibration of LRFD Resistance Factors for Design of Spread Footings and Embankments in Cohesive Soils at Serviceability Limit States, Missouri Department of Transportation, OR11.XXX, XXX pp. (in preparation)
Wyllie, D.C. (1999), Foundations on Rock, E & FN Spon, Second Edition, 401 pp.

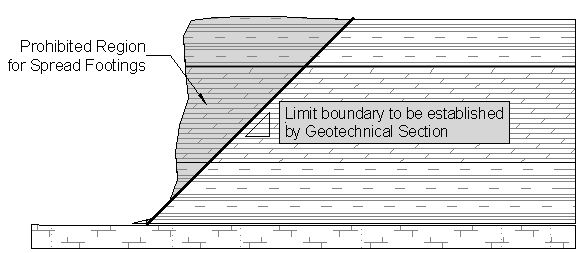




![{\displaystyle q_{n}=C_{f1}{\sqrt {s}}\cdot {\overline {q_{u}}}{\Bigg [}1+{\sqrt {{\frac {m}{\sqrt {s}}}+1}}{\Bigg ]}\leq 200ksf}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3af7f9d78e0dff3440db6bda4a272a1ef0607e9c)



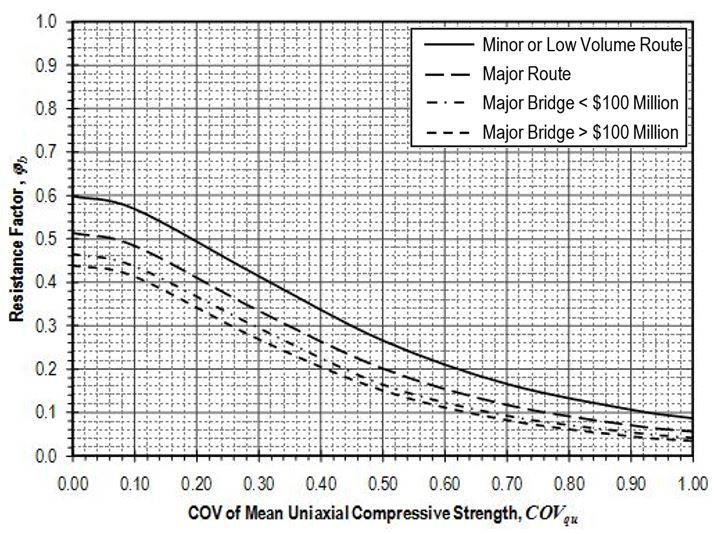





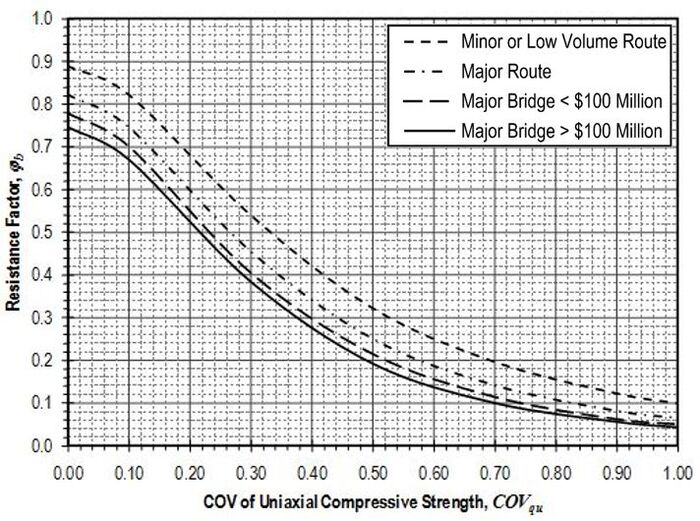






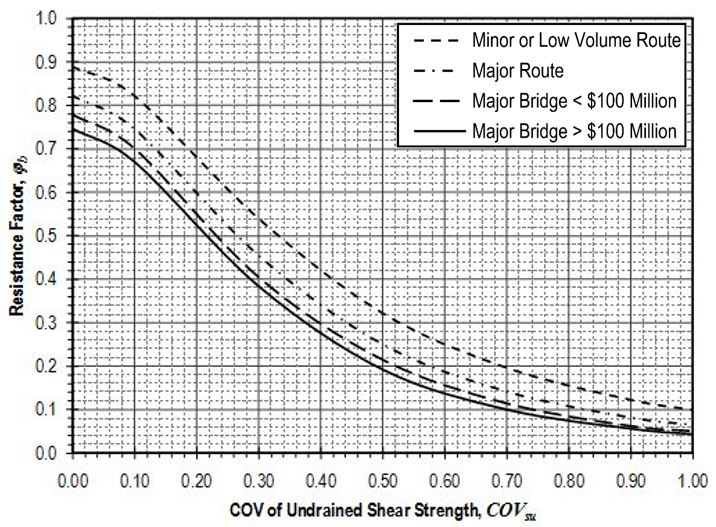






![{\displaystyle \delta _{R}={\frac {H_{0}}{1+e_{0}}}{\Bigg [}{\frac {c_{r}}{\phi _{r}}}log{\Big (}{\frac {\phi _{p}\sigma _{p}^{'}}{\sigma _{0}^{'}}}{\Big )}+{\frac {c_{c}}{\phi _{c}}}log{\Big (}{\frac {\sigma _{f}^{'}}{\phi _{p}\sigma _{p}^{'}}}{\Big )}{\Bigg ]}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/96a3e95b7a6c29a7ab7b907d5f3607658a44af90)
![{\displaystyle \delta _{R}={\frac {H_{0}}{1+e_{0}}}{\Bigg [}{\frac {c_{r}}{\phi _{r}}}log{\Big (}{\frac {\sigma _{f}^{'}}{\sigma _{0}^{'}}}{\Big )}{\Bigg ]}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ac2910954b17bd57557336e2c110eb7f4c8c05c6)
![{\displaystyle \delta _{R}={\frac {H_{0}}{1+e_{0}}}{\Bigg [}{\frac {c_{c}}{\phi _{c}}}log{\Big (}{\frac {\sigma _{f}^{'}}{\sigma _{0}^{'}}}{\Big )}{\Bigg ]}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e9b25994013b657e8b9140318239140afb9c9265)